矩形谱宽带激光中带宽诱导的焦开关现象

张笔雨; 彭润伍; 张伟

doi:10.7510/jgjs.issn.1001-3806.2017.01.028

矩形谱宽带激光中带宽诱导的焦开关现象

长沙理工大学物理与电子科学学院, 长沙 410114

基金项目:

长沙市科技计划重点资助项目 K1207107-11

详细信息

作者简介:
张笔雨(1991-), 男, 硕士研究生, 主要从事光电信息技术方面的研究

通讯作者:
彭润伍, E-mail:pengrunwu@163.com

中图分类号: O436
计量
- 文章访问数: 5
- HTML全文浏览量: 1
- PDF下载量: 5
出版历程
- 收稿日期: 2015-11-26
- 修回日期: 2015-12-10
- 发布日期: 2017-01-24

Bandwidth-induced focal switch in broadband laser with rectangular spectrum

Department of Physics and Electronic Science, Changsha University of Science and Technology, Changsha 410114, China

摘要

摘要: 为了研究频带宽度对激光光束传输和激光应用产生的影响，采用衍射积分推导了矩形谱宽带激光通过双焦色散透镜后的传输公式，利用数值计算研究了矩形谱宽带激光带宽诱导的焦开关现象，分析得到带宽对光强分布和焦开关的影响。结果表明，带宽是影响光强分布和焦开关形成的重要因素；带宽变化会导致光强分布中光强主极大从一个位置跃变到另一个位置，从而形成焦开关现象。该研究结果有助于进一步推动宽频带激光的应用。
- 激光光学 /
- 焦开关 /
- 双焦系统 /
- 带宽
Abstract: In order to research the effects of frequency bandwidth on the propagation and applications of broadband laser, the propagation formula of broadband laser with rectangular spectrum passing through a bifocal dispersion lens system was derived by using diffraction integral. Bandwidth-induced focal switch in broadband laser with rectangular spectrum is studied by numerical calculation. The effects of bandwidth on the intensity distribution and focal switch were analyzed. The results show that bandwidth is an important factor affecting intensity distribution and focal switch. Primary maximum intensity of the focused broadband laser can rapidly bounce from one place to another with the variety of bandwidth and then the focal switch is generated. The results are helpful for further applications of broadband laser.
- laser optics /
- focal switch /
- dual-focus system /
- bandwidth

HTML全文

引言

ALFANO等人在1970年首次获得谱宽范围在400nm~700nm的超连续谱光源^[1-2]。这类新光源在很多领域得到重要应用，JONES等人展示了其在频率计量以及在光相干断层摄影术和光通信等领域的应用^[3-5]。正因为此，宽带光源的产生和光源频带宽度对光束传输特性的影响也引起了人们极大的研究兴趣^[6-11]。早期MARTINEZ和CLIMENT发现, 当会聚球面波通过近轴超分辨率衍射屏时，会出现焦点位置跃变的现象，即所谓的焦开关^[12]。随后，这一现象得到广泛研究^[13-16]。已有的研究结果表明，焦开关受聚焦系统的菲涅耳数和光束参量等因素影响。然而直到近些年来，关于焦开关现象的研究仍局限于单色或准单色激光。为清楚宽频带光源中带宽对光强分布和焦开关的影响，本文中研究矩形谱宽带激光通过双焦色散透镜的传输特性及其带宽诱导的焦开关现象。首先从理论上推导了光强分布公式，然后给出了数值计算结果并讨论带宽对光强分布和焦开关的影响，最后对结果进行了总结。

1. 矩形谱宽带激光通过双焦色散透镜传输公式

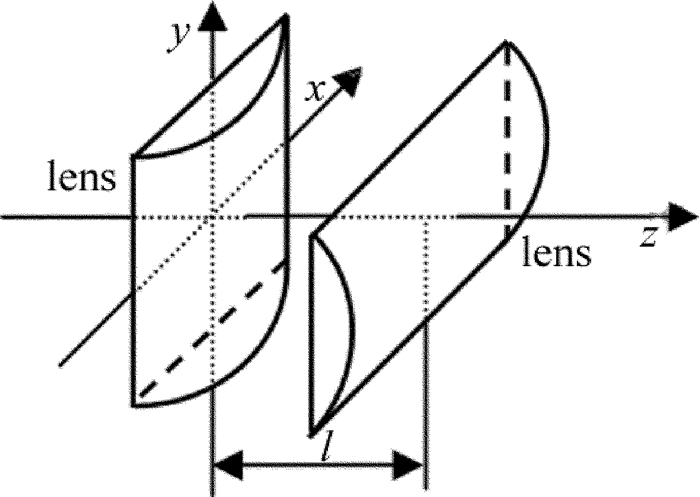
考虑由两个色散柱面透镜组成的双焦光学系统，如图 1所示, 图中l是两个柱面透镜之间的距离。x方向的焦距为f_x(λ)，y方向为f_y(λ)，且f_x>0, f_y>0，f_x(λ)和f_y(λ)与波长有关:

$\left\{ \begin{array}{l} {f_x}\left( \lambda \right) = {f_{0, x}}\frac{{{n_0} - 1}}{{n\left( \lambda \right) - 1}}\\ {f_y}\left( \lambda \right) = {f_{0, y}}\frac{{{n_0} - 1}}{{n\left( \lambda \right) - 1}} \end{array} \right.$

(1)

Figure 1. Schematic illustration of bifocal dispersion lens system

下载: 全尺寸图片幻灯片

式中, n₀, f_{0, x}和f_{0, y}分别是中心波长λ₀对应的折射率、x方向和y方向的焦距; n(λ)是与波长λ相关的折射率。考虑色散透镜为熔石英材质，其折射率为^[17]:

${n^2}\left( \lambda \right) = 1 + \sum\limits_{i = 1}^3 {} \frac{{{B_i}}}{{1 - \frac{{{\lambda _i}^2}}{{{\lambda ^2}}}}}$

(2)

式中, B₁=0.6961663, B₂=0.4079426, B₃=0.8974794, λ₁=0.0684043μm, λ₂=0.1162414μm，λ₃=9.896161μm。

考虑l=0的情况，则入射面到考察点z处的传输矩阵为:

$\left\{ \begin{array}{l} \left[ {\begin{array}{*{20}{c}} {{A_x}}&{{B_x}}\\ {{C_x}}&{{D_x}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {1 - \frac{z}{{{f_x}\left( \lambda \right)}}}&z\\ { - \frac{1}{{{f_x}\left( \lambda \right)}}}&1 \end{array}} \right]\\ \left[ {\begin{array}{*{20}{c}} {{A_y}}&{{B_y}}\\ {{C_y}}&{{D_y}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {1 - \frac{z}{{{f_y}\left( \lambda \right)}}}&z\\ { - \frac{1}{{{f_y}\left( \lambda \right)}}}&1 \end{array}} \right] \end{array} \right.$

(3)

根据Collins公式，宽带激光通过双焦透镜系统后在z处的场分布为:

$\begin{align} &E\left( x, y, z \right)=\frac{\text{exp}(\text{i}kz)}{\text{i}\lambda }\sqrt{\frac{1}{{{B}_{x}}{{B}_{y}}}}\times \\ &\iiint{{}}{{E}_{0}}({{x}_{0}}, {{y}_{0}}, z, \omega )\text{exp}\left[ -\frac{\text{i}k}{2{{B}_{x}}}({{A}_{x}}{{x}_{0}}^{2}-2{{x}_{0}}x+{{D}_{x}}{{x}^{2}}) \right]\times \\ &\text{exp}\left[ -\frac{\text{i}k}{2{{B}_{y}}}({{A}_{y}}{{y}_{0}}^{2}-2{{y}_{0}}y+{{D}_{y}}{{y}^{2}}) \right]\text{d}{{x}_{0}}\text{d}{{y}_{0}}\text{d}\omega \\ \end{align}$

(4)

式中, E₀(x, y, 0, ω)是入射面z=0处的场分布。考虑E₀(x, y, 0, ω)是可以分离为E₀(x, y, 0)S(ω)的简单情况，其中E₀(x, y, 0)和S(ω)分别是入射面z=0处的空间分布和频谱分布。假设空间分布E₀(x, y, 0)为高斯形，即:

${{E}_{0}}(x, y, 0)=\text{exp}\left[ -\left( \frac{{{x}^{2}}}{{{w}_{0, x}}^{2}}+\frac{{{y}^{2}}}{{{w}_{0, y}}^{2}~} \right) \right]$

(5)

式中, w_{0, x}, w_{0, y}分别是x方向和y方向的光束宽度。考虑激光的频谱为矩形谱:

$S\left( \omega \right)=\left\{ \begin{align} &1, (|\omega |\le \Delta \omega ) \\ &0, (|\omega |>\Delta \omega ) \\ \end{align} \right.$

(6)

式中, Δω=Δλω₀/λ₀是谱宽，ω₀是中心频率，Δλ是单位为nm的谱宽。

仅考虑轴上的场分布，因此对(4)式积分后得到:

$\begin{align} &E(0, 0, z)=\int_{-\infty }^{\infty }{{}}\frac{\text{i}k}{2z}\times \\ &\frac{S(\omega )\text{d}\omega }{\sqrt{\left\{ \frac{1}{{{w}_{0, x}}^{2}}+\frac{\text{i}k}{2}\left[ \frac{1}{z}-\frac{1}{{{f}_{x}}\left( \lambda \right)} \right] \right\}\left\{ \frac{1}{{{w}_{0, y}}^{2}}+\frac{\text{i}k}{2}\left[ \frac{1}{z}-\frac{1}{{{f}_{y}}(\lambda )} \right] \right\}}} \\ \end{align}$

(7)

由上式即可得到轴上的光强分布为:

$I\left( 0, 0, z \right)=E{{(0, 0, z)}^{2}}$

(8)

2. 计算分析和结果讨论

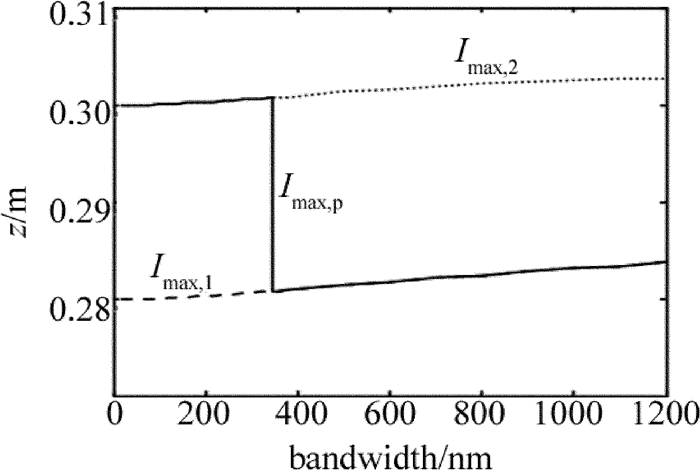
基于前面得到的(7)式和(8)式给出了数值计算例。图 2是宽带激光通过双焦透镜后的光强分布，计算参量为w_{0, x}=0.01m, w_{0, y}=0.02m, f_{0, x}=0.28m, f_{0, y}=0.3m, λ₀=800nm。分别用I_{max, 1}和I_{max, 2}表示光强分布中的第一和第二光强极大值，I_{max, p}表示光强主极大。从图中可以看到, 光强分布中有两个光强极大I_{max, 1}和I_{max, 2}，二者都随带宽变化。在带宽小于347nm时, I_{max, 2}为光强主极大I_{max, p}，如图 2a和图 2b所示。当带宽增大时，I_{max, 2}会减小，而I_{max, 1}则会增大。带宽等于347nm时, 两个光强极大相等，如图 2c所示。带宽继续增大时，I_{max, 1}超过I_{max, 2}从而成为光强主极大I_{max, p}，导致光强主极大位置发生了跃变，如图 2d和图 2e所示。由计算结果可知，随着带宽的增大，光强更趋于焦距较大的聚焦区域分布。与参考文献^[12-16]中针对单色和准单色光的研究内容相比，本文中的结果表明, 宽带激光中带宽也是影响光强分布的重要因素，带宽变化会诱导焦开关现象出现。

Figure 2. Axial intensity distributions of broadband laser passing through bifocal dispersion lens system

a—Δλ=50nm b—Δλ=160nm c—Δλ=347nm d—Δλ=800nm e—Δλ=1200nm

下载: 全尺寸图片幻灯片

图 3中给出了I_max，1, I_{max, 2}和I_{max, p}位置随带宽的变化。从图中可以看出，在带宽为347nm时, I_{max, 1}和I_{max, 2}分别位于0.2807m和0.3007m处，因为这时这两个光强极大相等，并且随带宽增大I_{max, 1}是增大的，因此光强主极大从0.3007m的位置处跃变到0.2807m处，在该处出现焦开关现象。图 2中还显示出带宽增大时I_{max, 1}和I_{max, 2}会远离对应的几何焦点，即出现了参考文献[18]中描述的正焦移现象。从图 3可以准确看出焦移量大小。当带宽从0nm增大到1200nm时，I_{max, 1}从0.2800m移动到0.2838m, 而I_{max, 2}从0.3000m移动到0.3027m，均有一定量的位置变化。这是宽带激光通过色散透镜系统一个特有的现象，光束的频谱和透镜的色散在其中起到重要作用。

Figure 3. Positions of the maximum intensity vs. bandwidth

下载: 全尺寸图片幻灯片

3. 结论

给出了矩形谱宽带激光通过双焦色散透镜后光强分布特点和随之出现的焦开关现象。带宽影响光强分布中两个光强极大，随带宽增大，其中一个光强极大变大而另一个减小，在二者相等时焦开关出现。同时二者随带宽增大不再位于对应的几何焦点处而是有所偏离。本文中的研究结果有助进一步了解宽带激光的传输特性，对宽带激光在光通信、光传感和光相干层析摄影术等领域的应用有参考意义。

Figure 1. Schematic illustration of bifocal dispersion lens system

下载: 全尺寸图片幻灯片

Figure 2. Axial intensity distributions of broadband laser passing through bifocal dispersion lens system

a—Δλ=50nm b—Δλ=160nm c—Δλ=347nm d—Δλ=800nm e—Δλ=1200nm

下载: 全尺寸图片幻灯片

Figure 3. Positions of the maximum intensity vs. bandwidth

下载: 全尺寸图片幻灯片

参考文献(18)

[1]	ALFANO R R, SHAPIRO S L. Observation of self-phase modulation and small-scale filaments in crystals and glasses[J]. Physics Review Letters, 1970, 24(11):592-594. DOI: 10.1103/PhysRevLett.24.592
[2]	ALFANO R R, SHAPIRO S L. Direct distortion of electronic clouds of rare-gas atoms in intense electric fields[J]. Physics Review Letters, 1970, 24(22):1217-1220. DOI: 10.1103/PhysRevLett.24.1217
[3]	JONES D J, DIDDAMS S A, RANKA J K, et al. Carrier-envelope phase control of femtosecond mode-locked lasers and direct optical frequency synthesis[J]. Science, 2000, 288(5466):635-639. DOI: 10.1126/science.288.5466.635
[4]	HSIUNG P, CHEN Y, KO T H, et al. Optical coherence tomography using a continuous-wave, high-power, Raman continuum light source[J]. Optics Express, 2004, 12(22):5287-5295. DOI: 10.1364/OPEX.12.005287
[5]	MORIOKA T, MORI K, SARUWATARI M. More than 100-wavelength-channel picosecond optical pulse generation from single laser source using supercontinuum in optical fibers[J]. Electronics Letters, 1993, 29(10):862-864. DOI: 10.1049/el:19930576
[6]	LI A P, ZHENG Y, ZHANG X F, et al. The supercontinuum generation in a photonic crystal fiber pumped at the anomalous dispersion region.Laser Technology, 2008, 32(1):50-52(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgjs200801013
[7]	AMENT C, POLYNKIN P, JEROME V M. Supercontinuum generation with femtosecond self-healing airy pulses[J]. Physics Review Letters, 2011, 107(24):243901. DOI: 10.1103/PhysRevLett.107.243901
[8]	REID D T. Ultra-broadband pulse evolution in optical parametric oscillators[J]. Optics Express, 2011, 19(19):17979-17984. DOI: 10.1364/OE.19.017979
[9]	CONFORTI M, BARONIO F, ANGELIS C D. Nonlinear envelope equation for broadband optical pulses in quadratic media[J]. Physical Review, 2010, A81(5):053841. http://d.old.wanfangdata.com.cn/OAPaper/oai_arXiv.org_1001.1874
[10]	PENG R W, LI L, LI Y J, et al. Intensity distribution of broadband laser with flattened-Gaussian mode passing through an aperture[J]. Laser Technology, 2013, 37(6):829-832(in Chinese) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgjs201306028
[11]	YUAN N, ZHANG W, WANG J, et al. Intensity distributions of a supercontinuum laser in an apertured dispersion lens[J]. Chinese Physics Letters, 2015, 32(3):034201. DOI: 10.1088/0256-307X/32/3/034201
[12]	MARTINEZ M, CLIMENT V. Focal switch:a new effect in low-Fresnel-number systems[J]. Applied Optics, 1996, 35(1):24-27. DOI: 10.1364/AO.35.000024
[13]	JI X L, LÜ B D. Focal shift and focal switch of flattened Gaussian beams in passage through an aperture bifocal lens[J]. IEEE Journal of Quantum Electronics, 2003, 39(1):172-178. DOI: 10.1109/JQE.2002.806204
[14]	DU X, ZHAO D. Focal shift and focal switch of focused truncated elliptical Gaussian beams[J]. Optics Communications, 2007, 275(2):301-304. DOI: 10.1016/j.optcom.2007.03.047
[15]	LIU W B, ZHONG M, HE H X, et al. Focal shift and focal switch of partially coherent annular beam focused by lens.Laser Technology, 2008, 32(3):259-261(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgjs200803014
[16]	MU G Q, WANG L, WANG X Q. Focal switch of cosine-squared Gaussian beams passing through an astigmatic lens.Laser Technology, 2011, 35(4):562-565(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgjs201104030
[17]	MALITSON I H. Interspecimen comparison of the refractive index of fused silica[J]. Journal of the Optical Society of America, 1965, 55(10):1205-1208. DOI: 10.1364/JOSA.55.001205
[18]	PENG R W, LI L, LI Y J, et al. Positive and negative focal shifts of an apertured supercontinuum laser with rectangular spectrum[J]. Optics Communications, 2013, 298/299:34-36. DOI: 10.1016/j.optcom.2013.02.051

施引文献(3)

期刊类型引用(2)

1.	吴金虎，彭润伍，谢鹏飞，唐俊龙，谢海情. 色散会聚透镜系统中的微米焦开关. 激光技术. 2021(03): 386-389 . 本站查看
2.	谢鹏飞，彭润伍，谢海情. 色散透镜系统中宽带厄米-高斯光束的焦移. 激光技术. 2019(03): 406-410 . 本站查看

其他类型引用(1)

资源附件(0)

图(3)

计量

文章访问数: 5
HTML全文浏览量: 1
PDF下载量: 5
被引次数: 3

引言
1. 矩形谱宽带激光通过双焦色散透镜传输公式
2. 计算分析和结果讨论
3. 结论

矩形谱宽带激光中带宽诱导的焦开关现象

作者简介:
张笔雨(1991-), 男, 硕士研究生, 主要从事光电信息技术方面的研究

通讯作者:
彭润伍, E-mail:pengrunwu@163.com

计量

Bandwidth-induced focal switch in broadband laser with rectangular spectrum