网站地图
-
甲烷(CH4)是一种易爆炸性气体，通常存在于气体混合物中[1-2]。它也是造成瓦斯爆炸的主要气体，当CH4体积分数达到4%~5%[3]时，就可能导致危险。由于缺乏高精度的检测装置，瓦斯爆炸每年在世界各地造成许多人死亡。因此，对CH4气体体积分数进行实时监测具有重要意义。近年来，随着对CH4体积分数传感器的研究越来越深入，许多新的检测结构被提出[4-9]，包括表面等离子体共振结构、周期光栅结构、金属氧化物催化、腔内增强吸收结构等。2015年，DHIVYA等人[4]研究了溅射功率对室温下反应直流磁控溅射沉积在玻璃衬底上的CdO薄膜性能的影响, 在100 ℃的工作温度下，该传感器能够实现0.0005%的CH4体积分数检测。2017年，YANG等人[6]研究了在聚丙烯酸-碳纳米管/盐酸聚丙烯胺纳米膜上吸收cryptophane-A-6me的高灵敏度光子晶体光纤长周期光栅CH4传感器, 当CH4体积分数从0增加到3.5%时，该传感器的灵敏度为107.8 nm，检测下限为0.0018。2019年，LIU等人[7]提出了一种基于液体渗透光子晶体光纤的高灵敏度温度补偿甲烷传感器，最高温度需达到59 ℃; 靠近核心区域的两个较大的孔被覆盖了甲烷敏感的复合膜，特定的包层气孔渗透到液体材料中形成新的缺陷通道; CH4体积分数在0~3.5%范围内，灵敏度可达2007 nm。虽然这些传感原理各有优势，但它们要么需要复杂的外部电流和电压条件，难以操作；要么高度依赖于温度，这反过来又加剧了气体爆炸的风险；要么结构复杂，加工难度大，因此，到目前为止，设计结构简单、检测精度高、对高温依赖性低的CH4传感器仍然是一个挑战。
光子自旋霍尔效应(photonic spin Hall effect，PSHE)是指线偏振光束入射到结构表面反射后，由于光束左旋和右旋圆偏振分量几何相位不同的影响，散射光场自旋分离的现象。PSHE是一种基于自旋轨道角动量耦合的光子效应，高度依赖于介质的折射率梯度。最初，PSHE中的自旋分裂位移非常微弱，通常仅在纳米尺度上，因此很难直接观察到。由于引入了弱测量技术[10-12]，自旋分裂位移增加了几个数量级，相对容易检测，这进一步增加了其实际应用的可能性，因此，它作为一种有趣的光学现象被广泛研究，如在光学物理[13]、高能物理[14]、超表面[15]等。
PSHE易受外界环境影响，这为精确测量技术提出了新的要求，在传感器设计中也更加受到重视。前人已经做了大量的研究来探索其传感特性[16-18]。2018年，ZHOU等人[10]提出了一种采用弱测量方法进行检测的PSHE折射率传感器, 采用表面等离子体共振技术设计了测量范围为1.33~1.335、灵敏度为1.088×105 μm/RIU(refractive index unit, RIU为折射率单位)的检测装置。同年，SHENG等人[19]也提出了一种高灵敏度的PSHE折射率传感器，其灵敏度高达3.16×10-5 μm/RIU，但是测量范围仅为3个折射率点。2020年，ZHANG等人[20]利用磁光PSHE效应实现了对低体积分数氯化钠溶液的测量, 基于磁光效应的非互易传输特性，传感器的测量范围为氯化钠体积分数的0~2%，灵敏度为2.9×104 μm/RIU。由此可见，前人已经提出了许多基于PSHE的传感器结构，并且体现出了PSHE与众不同的独特优势，但是迄今为止，似乎还没有关于CH4体积分数检测的报道。
本文中采用1维多层结构激发了尖锐的PSHE峰，多层结构由金属银、多孔硅以及甲烷敏感膜交替形成。由于非对称的排列方式打破了结构的宇称性，因此产生了单向的PSHE现象。PSHE被激发的角度位置以及峰值大小可以由气体孔隙率、周期数、金属层厚度以及甲烷敏感膜厚度进行调控。甲烷敏感膜的引入使得甲烷气体体积分数的折射率变化提高了两个数量级，这为检测提供了很大的便利。结果表明，该传感器可对CH4体积分数变化为0~3%时进行选择，并且灵敏度、质量因素和检测下限分别为29.6°，395和0.00012。
-
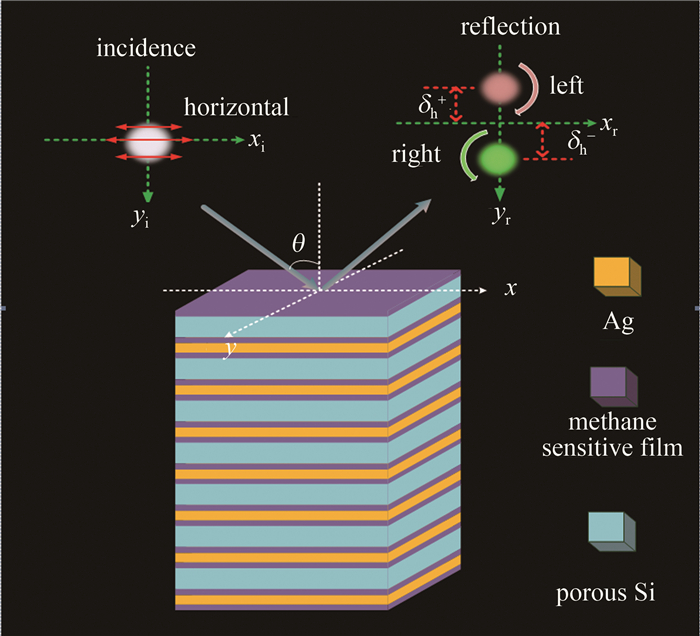
图 1是所提出的非互易光子自旋霍尔效应甲烷体积分数传感器的理论模型图。整体结构的拓扑排列形式为(ABAC)NA, 其中，电介质A层为甲烷敏感膜，B层为多孔硅porous Si, C层为金属银Ag，N为周期数，θ为入射角，xi和yi表示入射光的参考坐标系，xr和yr表示反射光的参考坐标系。
图 1 非互易PSHE甲烷体积分数传感器的理论模型图
Figure 1. Theoretical model diagram of a non-reciprocal PSHE methane volume fraction sensor
采用毛细管浸涂技术制备了一种紫外固化含氟硅(UV-curable fluoro-silicone, UVCFS)纳米膜[21]。温度和湿度对甲烷敏感膜的影响非常小。CH4气体的折射率接近空气，难以直接测量，且容易受到其它气体的干扰，因此检测精度较低。利用该敏感膜的特性，可将折射率变化现象扩大两个数量级，并可避免其它气体的干扰。甲烷敏感膜层响应时间低于60 s[22]。为了方便表示，甲烷体积分数用φCH4表示, 当φCH4为0~3%时，敏感膜的折射率呈线性变化, φCH4每增加1%，折射率降低0.0038，如果φCH4从0上升到3%，那么敏感膜的折射率neff从1.4478下降到1.4364[21]。
$ n_{\text {eff }}=1.4478-0.38 \varphi_{\mathrm{CH}_4} $
(1) 介质硅的折射率nSi可以描述为[23]:
$ n_{\mathrm{si}}=\sqrt{1+\frac{10.6684293 \lambda^2}{\lambda^2-0.301516485^2}+\frac{0.0030434748 \lambda^2}{\lambda^2-1.13475115^2}+\frac{1.54133408 \lambda^2}{\lambda^2-1104^2}} $
(2) 式中：λ为入射光波长。
利用Bruggeman的有效介质理论，多孔硅的介电常数np, Si可以被获得[23]:
$ n_{\mathrm{p}, \mathrm{Si}}=0.5 \sqrt{X+\sqrt{X^2+8 n_{\mathrm{Si}}{ }^2 n_{\text {gas }}{ }^2}} $
(3) $ X=3 P\left(n_{\text {gas }}{ }^2-{n_{\mathrm{Si}}}^2\right)+\left(2{n_{\mathrm{Si}}}^2-n_{\text {gas }}{ }^2\right) $
(4) 式中：np, Si、nSi和ngas分别是多孔硅、硅和孔隙内气体的折射率；P为气体孔隙率。
金属银Ag的介电常数εr采用德鲁德-洛伦兹模型表示[21]:
$ \varepsilon_{\mathrm{r}}=\varepsilon_{\infty}-\frac{\omega_{\mathrm{p}}^2}{\omega^2+\mathrm{i} \omega \gamma}-\frac{\mathit{\Omega}^2 \mathit{\Delta}}{\left(\omega^2-\mathit{\Omega}^2\right)+\mathrm{i} \mathit{\Gamma}} $
(5) 式中：ω=2πf为入射光的角频率；f=550 THz为频率；ε∞=2.4064为高频介电常数；ωp=2π×2214.6×1012 Hz为等离子体频率；γ=2π×4.8×1012 Hz为碰撞频率；Δ=1.6604是洛伦兹权重；Ω=2π×1330.1×1012 Hz为洛伦兹谐波强度；Γ=2π×620.7×1012为振动谱宽。
除了介电函数外，其它的参数可见表 1。表中, deff为甲烷敏感膜的厚度, dp, Si为多孔硅层的厚度, dAg为金属银层的厚度。
表 1 仿真所涉及的参数
Table 1. Parameters involved in the simulation
deff/nm dp, Si/nm dAg/nm N P 20 138 20 7 0.29 层与层之间的能量传播使用传输矩阵法进行计算。当一束线偏振光以折射率梯度反射到结构表面时，会分裂为左旋和右旋圆偏振光，反射光的水平偏振和垂直偏振的位移量可以表示为[20]:
$ \delta_{\mathrm{h}}^{+}=-\frac{\lambda}{2 {\rm{ \mathsf{ π}}}}\left[1+\frac{\left|r_s\right|}{\left|r_p\right|} \cos \left(\phi_s-\phi_p\right)\right] \cot \theta $
(6) $ \delta_{\mathrm{h}}^{-}=+\frac{\lambda}{2 {\rm{ \mathsf{ π}}}}\left[1+\frac{\left|r_s\right|}{\left|r_p\right|} \cos \left(\phi_s-\phi_p\right)\right] \cot \theta $
(7) $ \delta_{\mathrm{v}}{ }^{+}=-\frac{\lambda}{2 {\rm{ \mathsf{ π}}}}\left[1+\frac{\left|r_p\right|}{\left|r_s\right|} \cos \left(\phi_p-\phi_s\right)\right] \cot \theta $
(8) $ \delta_{\mathrm{v}}{ }^{-}=+\frac{\lambda}{2 {\rm{ \mathsf{ π}}}}\left[1+\frac{\left|r_p\right|}{\left|r_s\right|} \cos \left(\phi_p-\phi_s\right)\right] \cot \theta $
(9) 式中: 上标+和-分别表示左旋和右旋偏振分量; rs和rp为s波和p波的菲涅耳反射系数; ϕs和ϕp为反射相位; θ为入射角。
灵敏度S、品质因素Q、质量因素fm和检测下限D是衡量传感器的重要参数，性能突出的传感器对应着高S、高Q、高fm和低D。对应的定义如下:
$ S=\frac{{\Delta} \theta}{{\Delta} n_{\text {eff }}} $
(10) 式中: Δθ和Δneff为角度和折射率变化。
灵敏度S表征了传感器的谐振角度偏移量与待检测参量的变化量之间的对应关系[21, 23-24]。因此，灵敏度和角度的变化成正比，和有效折射率的变化成反比。当参数变化量相同时，检测到的谐振角度偏移量越大，传感器的灵敏度越高，也就越能够检测出越小的待测参量变化。
$ Q=\frac{\theta}{F} $
(11) 式中: F为半峰全宽[21]。品质因素Q是一个无量纲数值，表征微腔在时间上局域光的能力，与谐振腔内的损耗相关联，损耗越大，品质因素越低。该值的大小可以直观地从透射光谱中反映出来，透射峰越尖锐，微腔的品质因素越高，那么在读取谐振角度的大小时会更加准确。从定义上来看[21, 23]，品质因素和谐振角度的位置的变化成正比，和峰值半峰全宽的变化成反比。
$ f_{\mathrm{m}}=\frac{S}{F} $
(12) 质量因素fm被认为是指示传感器性能的重要参数。品质因数被定义为角度灵敏度与峰值半峰全宽的比值[21, 23]。质量因素和灵敏度的变化成正比，和峰值半峰全宽的变化成反比。通常，为了实现高质量因数性能传感器，灵敏度应尽可能高，而峰值半峰全宽应尽可能低。
$ D=\frac{\theta}{20 S Q} $
(13) 检测下限D反映的是传感器在检测分析物时能够检测到的分析物最小变化的能力。它表现为谐振角度与20倍的灵敏度和品质因素的乘积的比值[21, 23]，因此从公式上来看，检测下限和谐振角度的位置的变化成正比，和灵敏度以及品质因素的变化成反比。
-
在图 2中，当入射频率为550 THz时，产生了显著的PSHE现象，δh+值在入射角为67.42°的位置是3.92λ，在67.31°的位置为-0.41λ，而δv+值却是非常微弱的，仅仅在66.63°处产生了最大为0.07λ的位移，相比于水平偏振而言，垂直偏振的PSHE性能较差。此外，由于δh-值和δv-值与δh+值和δv+值仅在数值符号上相反，在具体数值大小上没有区别，因此，本文中不作特别分析。图 3中对水平偏振的非互易传输特性进行了研究。对于电磁波正向入射，在67.42°的位置产生了3.92λ的PSHE位移，而在67.31°的位置，产生了-0.41λ的位移。对于后向入射，几乎是不发生PSHE现象的。相比而言，这是很明显的PSHE单向传输特性，这主要得益于结构的非对称排列形式，巧妙的结构设计打破了结构的宇称性，从而激发了显著的非互易PSHE特征。
图 2 550 THz入射波激励下的水平偏振和垂直偏振位移量
Figure 2. Horizontal polarization and vertical polarization excited by 550 THz incident wave
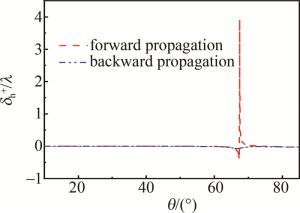
图 3 电磁波从相反的方向激励下对应的水平偏振分布
Figure 3. Corresponding horizontal polarization distribution phenomenon when the electromagnetic wave is excited from the opposite direction
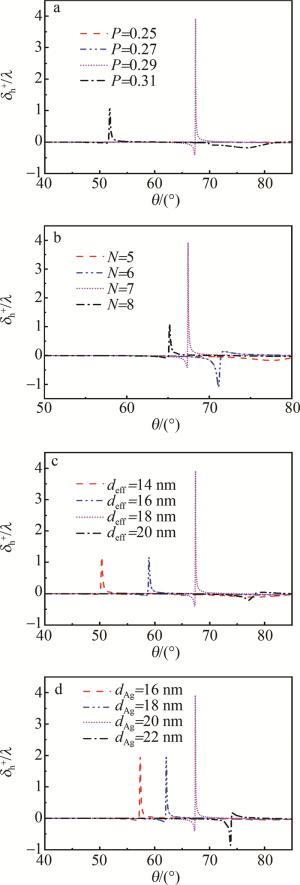
PSHE现象是在由多孔硅层、金属银层以及甲烷敏感膜层组成的多层结构中被激发的，因此3种材料的参数设置以及周期数也会对PSHE产生影响，图 4中对此进行了具体分析。图 4a中，当P分别代表不同的气体孔隙率时，PSHE效应差异显著, P=0.25时，不产生PSHE; 而当P取0.27，0.29或者0.31时，最大的PSHE位移量分别为-0.02λ，3.92λ，1.12λ，分别在83.91°，67.42°，51.8°的位置取得。随着气体孔隙率的增加，多孔硅的折射率也在提高，光波传输的光程随之增加，激励PSHE的结构条件发生了改变，因此PSHE的最大位移量呈现先增加后减小的趋势，而共振角则朝着小角度的方向移动。图 4b中，周期数N依次取值5，6，7，8时，最大位移量为-0.17λ，-1.09λ，3.92λ，1.09λ，相应的共振角度为77.61°，71.11°，67.42°，65.19°，类似地，周期数的增加使得位移量先逐渐增加，然后降低，并且共振角同样有着减小的趋势。图 4c中，如果甲烷敏感膜厚度deff以2 nm为间隔从14 nm增加到20 nm，那么最大位移量起初为1.16λ，接着保持在1.16λ，然后增加到3.92λ，最终缩减到-0.22λ，PSHE角度则一直在提高，依次取值50.36°，58.96°，67.42°，77.38°。图 4d中，银层厚度dAg的增加同样推动PSHE角度朝着大角度的方向移动，当dAg依次取值16 nm，18 nm，20 nm，22 nm时，最大位移角为57.39°，62.13°，67.42°，73.82°，相应地，最大位移量分别为1.98λ，1.99λ，3.92λ，-0.86λ。通过上述分析可知，结构参数和材料设计都会影响电磁波在结构中传播的光程分布，从而使得位移量和位移角产生偏移，这为PSHE的任意调控提供了理论基础，加大了其实际应用的可能性。
图 4 水平偏振特性随介质参数的影响
Figure 4. Horizontal polarization characteristics vary with medium parameters
通过对PSHE现象研究可以发现，在共振峰移动的过程中，始终呈现出十分尖锐的状态，这意味着PSHE峰具有极高的Q值，具有成为传感器的潜力。甲烷气体混合在空气中时，折射率和空气是非常接近的，很难区分，而当其通过多孔硅中的孔隙接触到甲烷敏感膜时，甲烷敏感膜的折射率就会发生显著的变化，从而为精确的判断提供机会。图 5a中，当甲烷气体体积分数为0时，敏感膜的折射率为1.4478，PSHE峰出现在67.42°，Q=898.9;当体积分数升为1%，敏感膜折射率为1.444，最大位移角为67.12°，Q=771.5;如果体积分数继续上升，分别变成2%和3%时，相应的其它指标依次为1.4402和1.4364，66.83°和66.53°，681.9和610.3。由分析可知，甲烷气体体积分数线性增加时，折射率线性降低。共振角度间隔为0.3°，0.29°，0.3°，变化也几乎是线性的，因此所提出的结构可用于甲烷气体体积分数检测。图 5b展示了甲烷气体体积分数从0上升到3%、折射率从1.4478降低到1.4364的连续状态。在变化过程中，共振峰始终是连续的，不存在跳变点，并且线性程度良好，因此在图 5c中，共振角度和气体体积分数变化被拟合。结果表明，拟合方程为：θ=-29.6°×φCH4+67.419°，方差R2=0.9999。在整个测量范围内，灵敏度为29.6°。图 5d中，φCH4=0时，fm和D分别为395和0.00012;φCH4=1%时，fm和D依次取值340和0.00015;φCH4=2%时，相应的指标为303和0.00017;φCH4=3%时，相应的指标为272和0.00018。由分析可知，随着甲烷气体体积分数的上升，折射率在下降，fm在降低，D在上升。fm最低值和最高值为272和395，D最低值和最高值为0.00012和0.00018，传感器最差也能够分辨0.018%的甲烷气体体积分数变化，这为甲烷气体体积分数的精细测量提供了新思路。
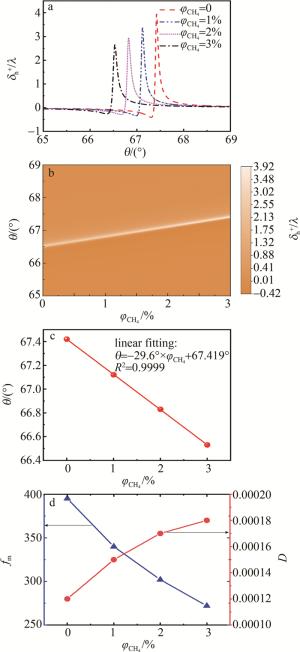
图 5 a—不同甲烷气体体积分数的位移分布情况现象 b—甲烷气体体积分数连续变化时的PSHE变化 c—甲烷体积分数和共振角度的线性拟合 d—特定的体积分数情况下，fm和D的分布情况
Figure 5. a—displacement distribution of different methane gas volume fraction b—PSHE phenomenon when methane gas volume fraction continuously changes c—linear fitting of methane volume fraction and resonance angle d—distribution of fm and D at specific volume fractions
图 6中，当周期数N分别为6，7，8时，测量范围为0~3%，拟合方差为0.9999，而其它指标略有不同。图 6a中，当N=6时，角度响应范围为71.11°~70.06°，灵敏度为34.9°，最高fm和最低D分别为118和0.00042。图 6b中，如果N=7，角度响应范围为67.42°~66.53°，灵敏度为29.6°，最高fm和最低D分别为395和0.00012。图 6c中，如果N=8，角度响应范围为65.193°~64.38°，灵敏度为27.2°，最高fm和最低D分别为153和0.00033。通过分析可知，随着周期数的增加，线性测量范围不变，灵敏度有所下降，拟合方差始终维持在较高水平，fm和D在本文中所选取的N=7时取得最优结果。
图 6 周期变化对线性拟合现象以及fm和D的分布的影响
Figure 6. Effects of periodic changes on the linear fitting phenomenon and the distribution of fm and D
-
利用非对称的多层堆叠结构激发了尖锐的非互易PSHE峰。结果表明，气体孔隙率、周期数、敏感膜厚度和金属厚度都会对PSHE的位移量和共振角度产生影响，其中，气体孔隙率和周期数的增加会使得共振角朝着小角度的方向移动，而敏感膜厚度和金属厚度的增强则会驱使共振角朝着大角度方向偏移。此外，借助于PSHE峰的高品质特性，研究了其在甲烷气体体积分数检测方面的应用。在0~3%的体积分数范围内，灵敏度为29.6°，质量因素fm的最小值和最大值分别为和272和395，检测下限D的最低值和最高值分别为0.00012和0.018%，这表明传感器最差也能够分辨0.018%的甲烷气体体积分数变化，这对于高精度的甲烷气体体积分数检测具有重要意义。在实验设计中，对于1维层状光子晶体的制备，可以采用刻蚀法。可在Si衬底上蚀刻所需的层数和相应层的厚度，然后填充结构所涉及的材料[25]。实验过程中，不可避免地会导致介质厚度出现偏差，故传感器的角度响应也会产生移动，而传感器依然可以进行体积分数检测，只是工作的角度范围产生偏移。因此，在传感器加工之后，首先要进行模拟测试，测定传感器的工作范围和性能指标，而不是单纯的依靠仿真结果。仿真结果也是必要的，因为实际的传感指标与仿真结果非常接近，仿真结果可以提供参考。
基于光子自旋霍尔效应的甲烷检测理论研究
Theoretical research of methane detection based on photonic spin Hall effect
-
摘要: 为了实现对甲烷体积分数的高精度检测, 采用多层结构激发了具有高品质因素的光子自旋霍尔效应现象。利用非对称的排列方式, 将甲烷敏感膜引入结构中, 通过敏感膜的折射率变化, 实现对甲烷体积分数的检测; 研究了气体孔隙率、周期数、金属厚度和敏感膜厚度对光子自旋霍尔效应的影响, 并采用传输矩阵法进行了数值分析。结果表明, 该传感器可对体积分数为0~3%、折射率变化为1.4364~1.4478的甲烷气体进行检测, 灵敏度为29.6°, 最高质量因素和最低检测下限分别为395和0.00012。该传感器结构简单、检测能力强, 为光学传感器的研究提供了新思路。Abstract: In order to realize the high precision detection of the volume fraction of methane, the photonic spin Hall effect phenomenon with high-quality factor is excited by a multilayer structure. In an asymmetric arrangement, a methane-sensitive film was introduced into the structure, and the volume fraction of methane could be detected by the change in the refractive index of the sensitive film. The effects of gas porosity, period number, metal thickness, and sensitive film thickness on the photon spin Hall effect were studied, and the transfer matrix method was used for numerical analysis. The results show that the sensor can detect methane gas with volume fraction of 0~3% (refractive index change is 1.4364~1.4478) with a sensitivity of 29.6°. The highest figure of merit and the lowest detection limit are 395 and 0.00012, respectively. The sensor has a simple structure and strong detection ability, which provides a new idea for the research of optical sensors.
-
图 1 非互易PSHE甲烷体积分数传感器的理论模型图
Figure 1. Theoretical model diagram of a non-reciprocal PSHE methane volume fraction sensor
图 2 550 THz入射波激励下的水平偏振和垂直偏振位移量
Figure 2. Horizontal polarization and vertical polarization excited by 550 THz incident wave
图 3 电磁波从相反的方向激励下对应的水平偏振分布
Figure 3. Corresponding horizontal polarization distribution phenomenon when the electromagnetic wave is excited from the opposite direction
图 4 水平偏振特性随介质参数的影响
Figure 4. Horizontal polarization characteristics vary with medium parameters
图 5 a—不同甲烷气体体积分数的位移分布情况现象 b—甲烷气体体积分数连续变化时的PSHE变化 c—甲烷体积分数和共振角度的线性拟合 d—特定的体积分数情况下，fm和D的分布情况
Figure 5. a—displacement distribution of different methane gas volume fraction b—PSHE phenomenon when methane gas volume fraction continuously changes c—linear fitting of methane volume fraction and resonance angle d—distribution of fm and D at specific volume fractions
图 6 周期变化对线性拟合现象以及fm和D的分布的影响
Figure 6. Effects of periodic changes on the linear fitting phenomenon and the distribution of fm and D
表 1 仿真所涉及的参数
Table 1. Parameters involved in the simulation
deff/nm dp, Si/nm dAg/nm N P 20 138 20 7 0.29 
下载: 导出CSV
-
[1] XIA Y, WAN J, XU L, et al. A room-temperature methane sensor based on Pd-decorated ZnO/rGO hybrids enhanced by visible light photocatalysis[J]. Sensors and Actuators, 2019, B304: 127334. [2] MULLER S A, DEGLER D, FELDMANN C, et al. Exploiting synergies in catalysis and gas sensing using noble metal-loaded oxide composites[J]. ChemCatChem, 2018, 10(5): 864-880. doi: 10.1002/cctc.201701545 [3] LIU L, LI G. A remote sensor for detecting methane based on palladium-decorated single walled carbon nanotubes[J]. Sensors, 2013, 13(7): 8814-8826. doi: 10.3390/s130708814 [4] DHIVYA P, PRASAD A K, SRIDHARAN M. Effect of sputtering power on the methane sensing properties of nanostructured cadmium oxide films[J]. Journal of Alloys and Compounds, 2015, 620: 109-115. doi: 10.1016/j.jallcom.2014.09.107 [5] LIU H, WANG M, WANG Q, et al. Simultaneous measurement of hydrogen and methane based on PCF-SPR structure with compound film-coated side-holes[J]. Optical Fiber Technology, 2018, 45: 1-7. doi: 10.1016/j.yofte.2018.05.007 [6] YANG J Ch, XIN C, RUI S, et al. High-sensitivity photonic crystal fiber long-period grating methane sensor with cryptophane-A-6Me absorbed on a PAA-CNTs/PAH nanofilm[J]. Optics Express, 2017, 25(17): 20258-20267. doi: 10.1364/OE.25.020258 [7] LIU H, WANG H R, ZHANG W, et al. High sensitive methane sensor with temperature compensation based on selectively liquid-infiltrated photonic crystal fibers[J]. Photonic Sensors, 2019, 9: 213-222. doi: 10.1007/s13320-019-0536-y [8] ZHENG K Y, ZHENG C T, LI J H, et al. Near-infrared methane sensor system using off-axis integrated cavity output spectroscopy with novel dual-input dual-output coupling scheme for mode noise suppression[J]. Sensors and Actuators, 2020, B308: 127674. [9] VUONG N M, HIEU N M, HIEU H N, et al. Ni2O3-decorated SnO2 particulate films for methane gas sensors[J]. Sensors and Actuators, 2013, B192: 327-333. [10] ZHOU X X, SHENG L J, LING X H. Photonic spin Hall effect enabled refractive index sensor using weak measurements[J]. Scientific Reports, 2018, 8: 1221. doi: 10.1038/s41598-018-19713-3 [11] KAVOKIN A, MALPUECH G, GLAZOV M. Optical spin hall effect[J]. Physical Review Letters, 2005, 95: 13660. [12] BLIOKH K Y, SMIRNOVA D, NORI F. Quantum spin Hall effect of light[J]. Science, 2015, 348(6242): 1448-1451. doi: 10.1126/science.aaa9519 [13] LUO H, ZHOU X, SHU W, et al. Enhanced and switchable spin Hall effect of light near the Brewster angle on refection[J]. Physical Review, 2011, A84: 043806. [14] WU Y D, SHENG L J, XIE L G, et al. Actively manipulating asymmetric photonic spin Hall effect with graphene[J]. Carbon, 2020, 166: 396-404. doi: 10.1016/j.carbon.2020.05.065 [15] SHALTOUT A, LIU J, KILDISHEV A, et al. Photonic spin hall effect in gap-plasmon metasurfaces for on-chip chiroptical spectroscopy[J]. Optica, 2015, 2(10): 860-863. doi: 10.1364/OPTICA.2.000860 [16] SRIVASTAVA A, SHARMA A K, PRAJAPATI Y K. On the sensitivity-enhancement in plasmonic biosensor with photonic spin Hall effect at visible wavelength[J]. Chemical Physics Letters, 2021, 774: 138613. doi: 10.1016/j.cplett.2021.138613 [17] LI N X, TANG T T, LI J, et al. Highly sensitive biosensor with graphene-MoS2 heterostructure based on photonic spin Hall effect[J]. Journal of Magnetism and Magnetic Materials, 2019, 484: 445-450. doi: 10.1016/j.jmmm.2019.04.003 [18] WANG Y H, JIN R C, LI J Q, et al. Photonic spin Hall effect by the spin-orbit interaction in a metasurface with elliptical nano-structures[J]. Applied Physics Letters, 2017, 110(10): 10190. [19] SHENG L J, XIE L G, LUO H L, et al. Sensitivity enhanced refractive index sensor by reducing the inflfluence of in-plane wavevector in photonic spin Hall effect[J]. IEEE Photonics Journal, 2018, 10(5): 6501209. [20] ZHANG P, TANG T, LUO L, et al. Magneto-optical spin Hall effect of light and its application in refractive index detection[J]. Optics Communications, 2020, 475: 126175. doi: 10.1016/j.optcom.2020.126175 [21] WAN B F, WANG Q Y, PENG H M, et al. A late-model optical biochemical sensor based on OTS for methane gas and glucose solution concentration detection[J]. IEEE Sensors Journal, 2021, 21: 21465-21472. doi: 10.1109/JSEN.2021.3103548 [22] LIU H, CHEN C, ZHANG Y Z, et al. A high-sensitivity methane sensor with localized surface plasmon resonance behavior in an improved hexagonal gold nanoring array[J]. Sensors, 2019, 19(21): 4803. doi: 10.3390/s19214803 [23] ZAKY Z A, AHMED A M, SHALABY A S, et al. Refractive index gas sensor based on the Tamm state in a one-dimensional photonic crystal: Theoretical optimization[J]. Scientific Reports, 2020, 10: 9736. doi: 10.1038/s41598-020-66427-6 [24] 陈强华, 丁锦红, 韩文远, 等. 光纤SPR传感器参数对折射率测量灵敏度的影响[J]. 激光技术, 2023, 47(3): 329-334. CHEN Q H, DING J H, HAN W Y, et al. Effect of optical fiber SPR sensor parameters on the sensitivity of refractive index measurement[J]. Laser Technology, 2023, 47(3): 329-334(in Chinese). [25] RENILKUMAR M, NAIR P. Properties of defect modes in geometrically chirped one-dimensional photonic crystals[J]. Optical Materials, 2011, 33(6): 853-858. doi: 10.1016/j.optmat.2011.01.008 -
点击查看大图
图(6) / 表(1)
计量
- 文章访问数: 2597
- HTML全文浏览量: 1503
- PDF下载量: 18
- 被引次数: 0
