FPGA-based adaptive fuzzy incremental PID control for fast steering mirror
-
摘要:
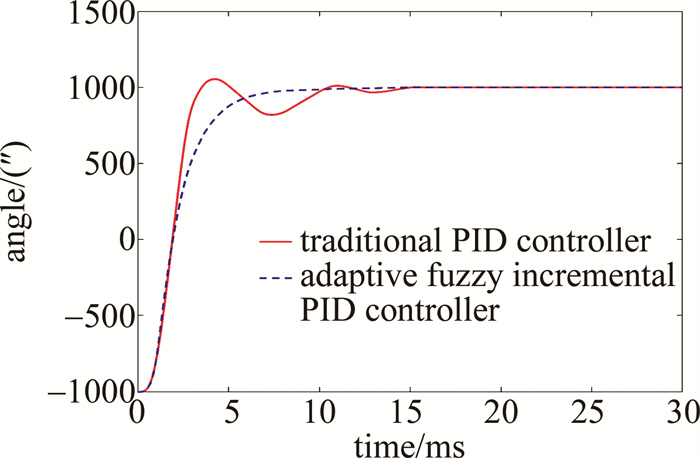
为了解决快速反射镜在传统比例-积分-微分(PID)控制下超调量大及稳定所需时间长的问题，引入自适应模糊算法优化控制器参数，提高控制性能。根据音圈电机快速反射镜的构成与工作原理，构建音圈电机快速反射镜的闭环控制模型，并针对模糊控制器中的隶属度函数及论域进行优化；通过现场可编程门阵列实现自适应模糊增量PID控制器，并进行了仿真及实物测试。结果表明，自适应模糊增量式PID控制器能使整体闭环过程“零超调”、“零振荡”，同时相比于传统PID控制器，系统稳定所需的时间缩短了17.5%，系统带宽提高了16.7%。该方法可有效提高快反镜的控制效果，可应用于其它需要高精度控制的系统当中。
-
关键词:
- 光学器件 /
- 快速反射镜 /
- 模糊比例-积分-微分控制 /
- 现场可编程门阵列 /
- 音圈电机
Abstract:To solve the problem of large overshooting and long time needed for stabilization of fast steering mirror (FSM) under traditional proportion-integration-differentiation (PID) control, adaptive fuzzy algorithm was introduced to optimize the control and improve the control performance. Based on the structure and working principle of the voice coil actuator (VCA) fast steering mirror, a closed-loop control model was constructed. The membership function and domain in the controller were optimized, and the adaptive fuzzy incremental PID controller was implemented through field-programmable gate array (FPGA). Simulations and physical tests were conducted. The results show that the adaptive fuzzy incremental PID controller can achieve "zero overshoot" and "zero oscillation" in the overall closed-loop process. At the same time compared with the traditional PID controller, the time required for system stabilization has been shortened by 17.5%, and the system bandwidth has been increased by 16.7%. This method can effectively improve the control effect of the fast steering mirror and can be applied to other systems requiring high-precision control.
-
引言
表面裂纹大量地存在于工程构件当中，如何快速准确地识别它们已成为无损检测的任务。普通的检测方法不适用于高温、高压、有毒[1]等恶劣环境，而激光超声无损检测凭借其非破坏、非接触[2-3]等优点克服了这种局限性，并且具有较高的精度，成为了无损检测的一个重要研究方向[4]。近些年来，随着计算机技术的飞速发展，计算机数值仿真有了越来越广泛的应用。关于激光超声无损检测的数值模拟已成为无损检测领域的一个热点[5-6]，而瑞利波进行无损检测的应用也越来越广[7-8]。通过模拟激光激发超声波的物理过程与超声波的传播过程，可以实现对表面裂纹的检测，从而对实验研究提供一种判定方法。
本文中将激光冲击作用进行简化，将激光的简化模型与激光的参量联系起来，利用ABAQUS软件模拟了激光激发出超声波的物理过程和超声波在材料表面进行传播的过程，并借此模型建立了表面裂纹深度与表面波信号特征量之间的联系，为激光超声检测表面裂纹深度提供了一种有效的方法。
1. 有限元模型
1.1 模型建立
建立一块各向同性且均匀的铝制薄板，铝板的长度为100mm，宽度为30mm，厚度为2mm，裂纹左边界距离板左端75.93mm。现有一束脉冲线源激光沿厚度方向照射到试样表面上，该问题可以简化成平面应变问题来分析, 如图 1所示。
为了精确模拟激光激发超声波的物理过程和超声波在表面的传播过程，网格的尺寸应不超过波长的1/4[9]。又考虑到计算效率，将网格尺寸取为0.040mm×0.013mm。在模型的左边界添加全约束。有限单元取平面应变单元CPE4R。用有限元来模拟超声波，模型必须要有足够大的几何尺寸才能避免来自边界的反射波对计算的影响，但这样会消耗大量的计算资源和计算时间。为了提高计算效率，同时也为了消除边界回波对表面波信号的干扰，在模型的左边界、右边界以及下边界使用无限单元，无限单元为CINPE4。超声波若在物体中能够完整的传播，需要有足够的时间，因此总时间长度取为5×10-5s，同时保证激光加载的精度，取时间步长为1×10-9s。
1.2 激光冲击作用的简化
为了使激光冲击作用的简化更接近于实际情况，体现激光在激发过程中的作用，需要将激光冲击作用与力作用相互联系。本文中考虑了激光的主要参量，即脉冲宽度、波长、能量、功率密度，从峰值功率密度、时间分布、空间分布3个角度将激光作用简化成力的作用。
激光波长λ=1064nm，脉冲宽度τ=10ns，激光作用面宽度d=0.6mm，单次脉冲能量E0=13.5mJ，峰值功率密度I0=E0τA=7.5×106W/cm2。其中，A为面积，线源脉冲激光在作用面上产生的峰值压力为[10]：
pmax (1) 式中，k为与材料有关的量，取k=2.3×10-7。
激光脉冲的空间分布函数[11]为：
\mathit{f}\left( r \right) = \exp \left( { - \frac{{{r^2}}}{{{r_0}^2}}} \right) (2) 式中, r0是激光辐照的半径, r为距激光作用面中心线的距离。
激光脉冲的时间分布函数[12]为：
g\left( t \right) = \alpha \frac{{8{t^3}}}{{{\tau ^4}}}\exp \left( { - \frac{{ - 2{t^2}}}{{{\tau ^2}}}} \right) (3) 式中，α为可由峰值压力确定的修正系数，取α=21，且载荷作用时间t取为脉冲宽度的3倍[13], 即载荷作用时间为30ns。
2. 数值结果与讨论
2.1 验证瑞利波
由于之后的研究是建立在瑞利表面波基础上的，所以有必要验证在模型上加载的脉冲是否能够产生瑞利表面波。一般瑞利表面波的验证是根据其性质来判断的。
首先在模型表面上离激励处较远的区域取3个点，得到如图 2所示的位移时间曲线。
图 2中1, 2, 3这3条曲线分别代表了离激励处越来越远的3个接收点的瑞利波信号。从图 2可以看出，在离激励作用位置较远的区域上，各节点的相对幅值的差距变小了，波形在传播的过程中将基本不变，此时产生了瑞利波，此点符合瑞利表面波的性质[14]。
然后，在模型不同深度的位置取3个点，得到如图 3所示的位移时间曲线。
图 3中4, 5, 6这3条曲线分别代表了深度越来越大的3个接收点的表面波信号。从图 3可以看出，随着深度的增加，节点振动的位移幅值逐渐衰减，此点符合瑞利表面波的衰减效应。
由此看出，简化后的激光冲击作用的确可以产生瑞利表面波。
2.2 表面裂纹深度对表面波信号的影响
在表面上距裂纹左边界10mm处取信号的接收点，同时裂纹深度分别为0μm，230μm，360μm时，接收点处瑞利波信号的位移时间曲线如图 4所示。
从图 4得知，当材料表面没有裂纹时，接收点处信号并没有出现瑞利反射回波。当材料表面存在裂纹时，接收点处信号出现了较强的瑞利反射回波。并且随着裂纹深度的增加，瑞利波信号的峰值呈显著增加的状态。因此这可以成为实验判断表面裂纹是否存在的主要依据。
图 5中给出了瑞利反射波的幅值与裂纹深度之间的关系。可以看出，裂纹深度在0μm~260μm时，瑞利波的峰值随着深度的增加而显著增加；裂纹深度在260μm~420μm时，瑞利波的峰值随着深度的增加而缓慢增加；裂纹深度在420μm以上时，瑞利波的峰值随着深度的增加而基本保持不变。这一变化趋势[15]的原因是瑞利波的能量分布主要集中在表面以下、一个波长的深度范围内，随着裂纹深度的增加，瑞利波被裂纹阻挡，发生反射的能量成分增长，回波的幅值也随深度的增加而增加。当裂纹深度超过一个波长量级时，深度的增加将仅会对反射的能量成分产生微小的作用，此时回波的幅值会随深度增加而产生微小的浮动。这一趋势进一步验证了有限元模型的正确性，但同时也表明了不能简单地根据瑞利波的峰值来判断裂纹深度。
![Figure 5. Peak values variation of Rayleigh wave signals with different surface crack depth]()
Figure 5. Peak values variation of Rayleigh wave signals with different surface crack depth为了使瑞利波的位移时间曲线能够反映与裂纹深度的关系，取反射波的正向峰值与下一次最大正向位移所对应的时间(t1与t2如图 6所示)的比值Δ=t2/t1为指标。图 7显示了该指标与裂纹深度之间的关系。
图 7中的黑点为通过上述模型计算出来的比值，直线为拟合直线。从图 7可以清晰地看到，时间比值Δ与裂纹深度之间存在着近似线性的关系，并且线性相关系数为98.9%。通过这种关系，可以通过实验测量进而得到表面裂纹的深度。
3. 结论
本文中建立了激光超声无损检测表面裂纹深度的有限元数值模型，考虑了激光工作参量将激光冲击作用等效成脉冲载荷作用，得到了性能良好的瑞利波。与传统的有限元模拟超声波的方法相比，引入无限单元能缩小模型尺寸，提高计算效率，并且也能有效消除边界反射波对计算的影响。模拟结果表明, 材料存在表面裂纹时, 接收点处会有明显的反射回波，借此可以判断材料表面是否存在裂纹。并且瑞利反射波的正向峰值与下一次最大正向位移所对应的时间比值Δ和裂纹深度之间存在线性关系。利用这一关系，可以为激光超声无损检测的实验测量提供一种有效方法。
-
![]()
图 2 自适应模糊增量式PID控制器的系统框图
Figure 2. System block diagram of adaptive fuzzy incremental PID controller
![]()
图 6 自适应模糊增量式PID控制器及传统PID控制器框图
Figure 6. Block diagram of adaptive fuzzy incremental PID controller and conventional PID controller
![]()
图 7 自适应模糊增量式PID控制器和传统PID控制器的控制效果仿真图
Figure 7. Simulation of control effect of adaptive fuzzy incremental PID controller and conventional PID controller
![]()
图 8 自适应模糊增量式PID控制器代码运行结果
Figure 8. Code run results of adaptive fuzzy incremental PID controller
![]()
图 9 自适应模糊增量式PID控制器和传统PID控制器的阶跃测试结果
Figure 9. Step test results of adaptive fuzzy incremental PID controller and conventional PID controller
![]()
图 10 带宽测试结果
a—传统PID控制器b—自适应模糊增量式PID控制器
Figure 10. Results of bandwidth test
a—conventional PID controller b—adaptive fuzzy incremental PID controller
表 1 自适应模糊增量式PID控制器和传统PID控制器的阶跃测试数据
Table 1 Step test data of adaptive fuzzy incremental PID controller and conventional PID controller
controller overshooting/ % time required for stabilization/ms number of oscillations traditional PID controller 5 10.3 2 adaptive fuzzy incremental PID controller 0 8.5 0 
下载: 导出CSV
-
[1] KLUK D J, BOULET M T. TRUMPERD L. A high-bandwidth, high-precision, two-axis steering mirror with moving iron actuator[J]. Mechatronics, 2012, 22(3): 257-270. DOI: 10.1016/j.mechatronics.2012.01.008
[2] 王震, 程雪岷. 快速反射镜研究现状及未来发展[J]. 应用光学, 2019, 40(3): 373-379. WANG Zh, CHENG X M. Research progress and development trend of fast steering mirror[J]. Journal of Applied Optics, 2019, 40 (3): 373-379(in Chinese).
[3] 周倩, 张兵, 李志俊, 等. 光电跟踪系统的区间2型模糊滑模控制方法[J]. 激光技术, 2023, 47(3): 293-300. ZHOU Q, ZHANG B, LI Zh J, et al. Interval type-2 fuzzy sliding mode control method of electro optical tracking system[J]. Laser Technology, 2023, 47(3): 293-300(in Chinese).
[4] WANG Y K, LI D Y, WANG R, et al. High-bandwidth fine tracking system for optical communication with double closed-loop control method[J]. Optical Engineering, 2019, 58(2): 026102.
[5] 王毅刚, 刘力双, 康登魁, 等. 用于深空探测快速反射镜的电磁驱动[J]. 激光技术, 2023, 47(2): 214-219. DOI: 10.7510/jgjs.issn.1001-3806.2023.02.009 WANG Y G, LIU L Sh, KANG D K, et al. Research on electromagnetic drive of fast steering mirror for deep space detection[J]. Laser Technology, 2023, 47(2): 214-219(in Chinese). DOI: 10.7510/jgjs.issn.1001-3806.2023.02.009
[6] IBRAHEEM K I, WAMEEDH R A. On the improved nonlinear tracking differentiator based nonlinear PID controller design[J]. International Journal of Advanced Computer Science and Applications, 2016, 7(10): 234-241.
[7] 艾志伟, 谭毅, 吴琼雁, 等. 改进根轨迹的快速反射镜控制参量设计[J]. 激光技术, 2017, 41(4): 558-561. AI Zh W, TAN Y, WU Q Y, et al. Design of control parameters for fast steering mirrors by improving root locus[J]. Laser Technology, 2017, 41(4): 558-561(in Chinese).
[8] KUMAR M, RAWAT T K. Optimal design of FIR fractional order di-fferentiator using cuckoo search algorithm[J]. Expert Systems with Applications, 2015, 42(7): 3433-3449. DOI: 10.1016/j.eswa.2014.12.020
[9] 曹洪瑞, 刘永凯, 张淑梅. 基于快速反射镜的自适应控制算法研究[J]. 传感器与微系统, 2017, 36(1): 16-19. CAO H R, LIU Y K, ZHANG Sh M. Adaptive control algorithm research based on fast steering mirror[J]. Transducer and Microsystem Technologies, 2017, 36(1): 16-19(in Chinese).
[10] 刘献之, 贾建军, 黄迪山, 等. 光自闭环快反镜的控制器设计[J]. 仪表技术与传感器, 2017(3): 37-41. LIU X Zh, JIA J J, HUANG D Sh, et al. Design of optical self closed loop fast steering mirror controller[J]. Instrument Technique and Sensor, 2017(3): 37-41(in Chinese).
[11] WANG R, WANG Y K, JIN Ch B, et al. Demonstration of horizontal free-space laser communication with the effect of the bandwidth of adaptive optics system[J]. Optics Communications, 2019, 431: 167-173. DOI: 10.1016/j.optcom.2018.09.038
[12] WANG Zh X, ZHANG B, LI X T, et al. Study on application of model reference adaptive control in fast steering mirror system[J]. Optik, 2018, 172: 995-1002. DOI: 10.1016/j.ijleo.2018.07.095
[13] 舒郁, 陈青山, 夏润秋, 等. 光电精跟踪系统音圈电机快反镜的预先修正控制[J]. 激光技术, 2023, 47(6): 811-815. SHU Y, CHEN Q Sh, XIA R Q, et al. Pre-amending and controllment of voice coil actuator fast steering mirror of opto-electronic fine tracking system[J]. Laser Technology, 2023, 47(6): 811-815(in Chinese).
[14] GAO Q, FENG G, WANG Y, et al. Universal fuzzy models and universal fuzzy controllers for stochastic non-affine nonlinear systems[J]. IEEE Transactions on Fuzzy Systems, 2013, 21(2): 328-341. DOI: 10.1109/TFUZZ.2012.2213823
[15] ZHOU Y, WU Y M, YANG T H. Study on electric actuator control system[C]//2019 Chinese Control and Decision Conference (CCDC). New York, USA: IEEE Press, 2019: 865-870.
[16] 王晓侃, 王亮. 基于MATLAB的模糊PID参数自整定控制器设计与研究[J]. 新技术新工艺, 2016(11): 26-28. WANG X K, WANG L. Design and research based on fuzzy PID-parameters self-tuning controller with MATLAB[J]. New Technology & New Process, 2016(11): 26-28(in Chinese).
[17] 石坤. 基于Matlab/Simulink的模糊主动控制仿真[J]. 电子测试, 2018(23): 5-7. SHI K. Simulation experiment on fuzzy controller based on Matlab/Simulink[J]. Electronic Test, 2018(23): 5-7(in Chinese).
[18] WANG H Q, CHEN B, LIU X P, et al. Robust adaptive fuzzy tracking control for pure-feedback stochastic nonlinear systems with input constraints[J]. IEEE Transactions on Cybernetics, 2013, 43(6): 2093-2104. DOI: 10.1109/TCYB.2013.2240296
[19] 宋超, 曹翱, 温家玺. 自适应模糊PID控制器的设计及MATLAB仿真[J]. 现代制造技术与装备, 2018, 260(7): 203-205. SONG Ch, CAO A, WEN J X. Design of adaptive fuzzy PID controller and MATLAB simulation[J]. Modern Manufacturing Techno-logy and Equipment, 2018, 260(7): 203-205(in Chinese).
[20] 李强. 基于模糊控制理论的PID闭环控制系统的研究与设计[J]. 现代电子技术, 2015, 38(22): 131-134. LI Q. Research and design of PID closed-loop control system based on fuzzy control theory[J]. Modern Electronics Technique, 2015, 38(22): 131-134(in Chinese).
[21] ZHAO Ch, LIU J G, TANG Y. Design and simulation of fuzzy self-adaption PID three closed loop control based on Simulink[J]. Digi-tal Technology & Application, 2019, 37(10): 1-4(in Chinese).
-
期刊类型引用(14)
1. 张彦杰，莫海峰，于程豪，张超，侯文静，张忠，杜晓钟，邬宇. 弧形表面缺陷的激光超声检测方法研究. 应用激光. 2023(02): 107-115 . 
百度学术
2. 陆思烨，张兴媛，金桢玥. 声表面波反射分量对金属表面裂纹深度的定量研究. 应用激光. 2023(08): 139-150 . 百度学术
3. 刘孝义，冯路，董斌，刘攀，裴平. 孔内充装液态CO_2相变致裂技术及应用. 青海交通科技. 2023(05): 80-89 . 百度学术
4. 扈康佳，侯贺骞，高浩岩，李宗强，张靖轩，邸志刚. 激光超声对橡胶材料表面损伤的仿真研究. 电声技术. 2022(04): 6-9 . 百度学术
5. 陈超，张兴媛，宋大成，王啸伟. 激光超声检测材料内部缺陷的数值模拟. 智能计算机与应用. 2020(05): 89-92+97 . 百度学术
6. 左欧阳，武美萍，唐又红. 基于COMSOL的激光超声裂纹检测数值模拟研究. 激光与红外. 2020(10): 1164-1171 . 百度学术
7. 唐又红，左欧阳，武美萍. 激光超声裂纹深度定量检测研究. 应用激光. 2020(06): 1144-1152 . 百度学术
8. 陈春明，战宇，刘常升，方奔. 激光超声测量T800/QY9511复合材料工程常数的有限元模拟. 激光技术. 2019(01): 88-92 . 本站查看
9. 王玉庆，王云霞，马世榜. 基于衍射横波的裂纹激光超声检测方法. 激光技术. 2019(04): 110-114 . 本站查看
10. 姬冠妮，王亚亚，史二娜. 激光超声检测技术的材料表面微小损伤检测. 激光杂志. 2019(09): 65-68 . 百度学术
11. 冯湾湾，金磊，赵金峰，潘永东. 激光激发横波作用垂直裂纹侧面后的模式转换. 激光技术. 2018(04): 487-493 . 本站查看
12. 徐志祥，黄建华，王铮恭，黄义敏，王雨. 激光超声检测带涂层金属表面裂纹的数值研究. 激光技术. 2018(06): 801-805 . 本站查看
13. 陶程，殷安民，应志奇，王煜帆，束学道，彭文飞. 不同温度下表面微缺陷的声表面波定量分析. 激光与光电子学进展. 2018(12): 370-378 . 百度学术
14. 孙继华，赵扬，南钢洋，马健，刘帅，王启武，巨阳. 钢轨轨腰缺陷激光-电磁超声检测及信号处理. 激光杂志. 2017(12): 22-25 . 百度学术
其他类型引用(12)
计量
- 文章访问数: 6
- HTML全文浏览量: 0
- PDF下载量: 5
- 被引次数: 26