New optical image encryption technology based on grating filter
-
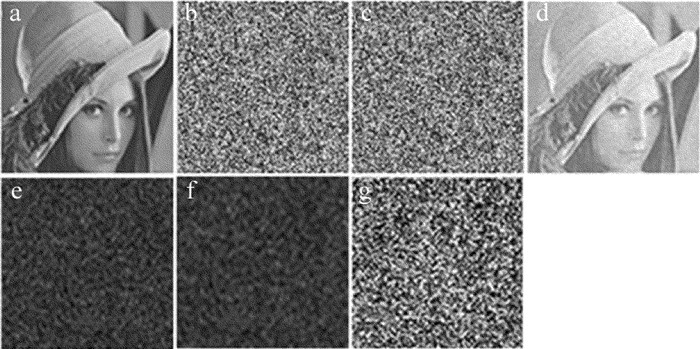
摘要: 为了提高干涉加密技术的光学处理速度和光学实现的可行性，基于干涉原理的加密技术的思想，结合光栅滤波器的图像处理方法，研究了基于光栅滤波器的4f系统双图像光学加密技术。该技术利用已成熟的基于光栅滤波的图像相加减4f系统，将两个待加密图像转化为两个实值的白噪声，理论模拟验证了该方法的可行性和有效性。结果表明，该方法简单实用，易于光学实现并具有很高的安全性。Abstract: In order to improve optical processing speed and the feasibility of optical realization in interference encryption technology, based on the idea of interference encryption principle and image processing method of grating filter, 4f system double image optical encryption technology based on grating filter was designed. The mature image additiion and subtraction 4f system based on grating filtering was used to transform two encrypting images into two real values of white noise. The feasibility and effectiveness of the method were verified by theoretical simulation. The results show that the method is simple, practical, safe and easy to implement.
-
Keywords:
- information optics /
- optical encryption /
- image encryption /
- grating filter /
- interference
-
-
![]()
Figure 2. a—the image to be encrypted b—the encrypted image o1 c—the encrypted image o2 d—decryption result with correct key e—decryption result with wrong key R1 f—decryption result with wrong key R2 g—decryption result with wrong key R3
-
[1] MATOBA O, JAVIDI B. Encrypted optical storage with wavelength-key and random phase codes[J]. Applied Optics, 1999, 38(32):6785-6790. DOI: 10.1364/AO.38.006785
[2] MATOBA O, JAVIDI B. The keys to holographic data security[J].IEEE Circuits and Devices Magazine, 2000, 16(5):8-15. DOI: 10.1109/101.876899
[3] XU G X, XU Sh Q, GUO X J, et al. Image compression-encryption algorithm combined DCT transform with DNA operation[J]. Laser Technology, 2015, 39(6):460-463(in Chinese). http://www.opticsjournal.net/abstract.htm?aid=OJ151130000298WtZv3y
[4] PAN T G, LI D Y. A novel image encryption using Arnold cat[J]. International Journal of Security and its Application, 2013, 7(5):377-386. DOI: 10.14257/ijsia
[5] XI S X, WANG X L, SONG L P, et al. Experimental study on optical image encryption with asymmetric double random phase and computer-generated hologram[J]. Optics Express, 2017, 25(7):8212-8222. DOI: 10.1364/OE.25.008212
[6] XIAO Y L, LIU Q, YUAN Sh. Research on optical images encryption system based on fresnel zone[J]. Laser Technology, 2009, 33(4):433-436(in Chinese).
[7] ZHANG H Zh, YAO M, LEI P. Research of image processing method of far-field laser spots[J]. Laser Technology, 2013, 37(4):460-463(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-JGJS201304011.htm
[8] REFREGIER P, JAVIDI B. Optical image encryption based on input plane and Fourier plane random encoding[J]. Optics Letters, 1995, 20(7):767-769. DOI: 10.1364/OL.20.000767
[9] JAVIDI B, CARNICER A, YAMAGUCHI M, et al. Roadmap on optical security[J]. Journal of Optics, 2016, 18(8):083001. DOI: 10.1088/2040-8978/18/8/083001
[10] UNNIKRISHNAN G, SINGH K. Double random fractional Fourier domain encoding for optical security[J]. Optical Engineering, 2000, 39(11):2853-2859. DOI: 10.1117/1.1313498
[11] MATOBA O, JAVIDI B. Encrypted optical storage with wavelength-key and random phase codes[J]. Applied Optics, 1999, 38(32):6785-6790. DOI: 10.1364/AO.38.006785
[12] GOODMAN J W, LAWRENCE R W. Digital image formation from electronically detected holograms[J]. Applied Physics Letters, 1967, 11(3):77-79. DOI: 10.1063/1.1755043
[13] CARNICER A, MONTES-USATEGUI M, ARCOS S, et al. Vulnerability to chosen cyphertext attacks of optical encryption schemes based on double random phase keys[J]. Optics Letters, 2005, 30(13):1644-1646. DOI: 10.1364/OL.30.001644
[14] FRAUEL Y, CASTRO A, NAUGHTON T J, et al. Resistance of the double random phase encryption against various attacks[J]. Optics Express, 2007, 15(16):10253-10265. DOI: 10.1364/OE.15.010253
[15] CHEN L F, ZHAO D M. Optical image encryption based on fractional wavelet transform[J]. Optical Communications, 2005, 254(4/6):361-367. http://www.sciencedirect.com/science/article/pii/S0030401805005274
[16] GLUCKSTAD J, MOGENSEN P C. Phase-only optical encryption[J]. Optics Letters, 2000, 25(8):566-568. DOI: 10.1364/OL.25.000566
[17] DARIA V R, SINZINGER S, GLUCKSTAD J. Phase-only optical decryption in a planar integratedmicro-optics system[J]. Optcal Engineering, 2004, 43(10):2223-2227. DOI: 10.1117/1.1782613
[18] MOGENSEN P C, GLUCKSTAD J. A phase-based optical encryption system with polarisation encoding[J]. Optical Communications, 2000, 173(1/6):177-183. http://www.sciencedirect.com/science/article/pii/S0030401899006331
[19] PENG X, CUI Z, TAN T. Information encryption with virtual-optics imaging system[J]. Optical Communications, 2002, 212(4/6):235-242. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ024816217/
[20] ZHANG Y, WANG B. Optical image encryption based on interfe-rence[J]. Optics Letters, 2008, 33(21):2443-2445. DOI: 10.1364/OL.33.002443
[21] JABLOWSKI D P, LEE S H. Restoration of degraded images by compo site gratings in a coherent optical prcessor[J]. Applied Optics, 1973, 2(7):1703-1712. http://www.opticsinfobase.org/ao/abstract.cfm?uri=ao-12-7-1703
下载:
计量
- 文章访问数: 5
- HTML全文浏览量: 1
- PDF下载量: 7