Generation of terahertz vortex beams base on metasurface antenna array
-
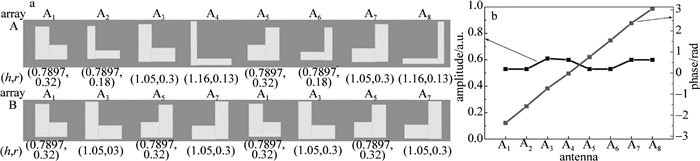
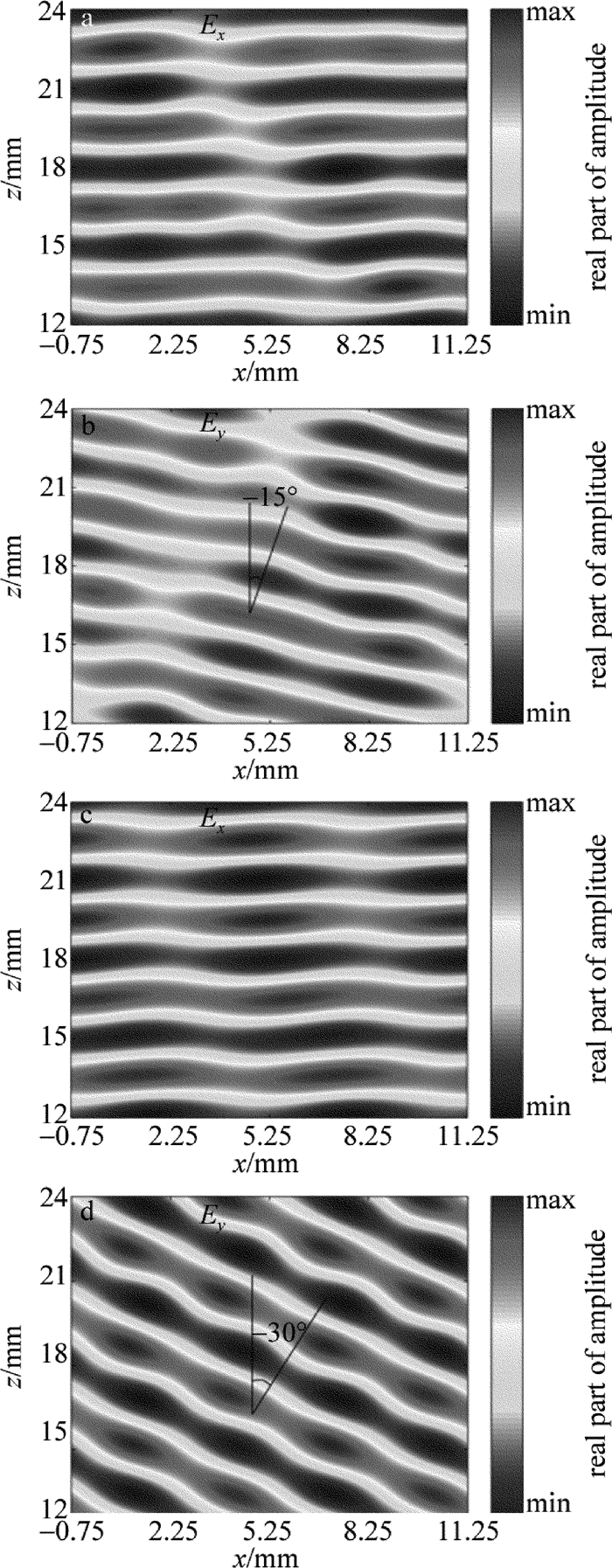
摘要: 为了研究1阶和2阶模式下的非连续性相位L型天线的超表面阵列特性，采用异常透射的散射场理论，设计了一种L型天线结构，控制天线的几何参量，选取阵列单元组，使得其覆盖相位超过2π。并根据不同的拓扑荷，设计1阶和2阶涡旋相位板，产生不同阶数涡旋光束。结果表明，用太赫兹线偏光垂直入射时，天线单元垂直偏振透射方向的模拟仿真效率达到55%左右；相位覆盖0~2π和0~4π时，其线性阵列的异常透射角不同，分别为-14.7°和-30°，其结果与广义斯涅耳定理一致。此研究对太赫兹涡旋光束的器件研究有重要的应用价值。Abstract: In order to study characteristics of metasurface array of discontinuous phase L-shaped antenna in the 1st and 2nd order modes, by using the theory of scattering field with anomalous transmission, a L-shaped antenna structure was designed. By controlling the geometric parameters of the antenna and selecting the array element group, the coverage phase exceeded 2π. According to different topological charges, the vortex phase plates of the 1st order and the 2nd order were designed to produce different order vortex beams. The simulation results show that the simulation efficiency of the vertical polarization direction of the antenna element is about 55% when the incident wave is polarized vertically. When the phase covered 0~2π and 0~4π, the anomalous transmission angles of the linear array are different, -14.7° and -30° respectively. The results are consistent with the generalized Fresnel theory. This study has the important application value in the research of terahertz vortex beam devices.
-
Keywords:
- scattering /
- terahertz /
- metasurface /
- anomalous transmission /
- vortex beam
-
-
![]()
Figure 1. a—2-D graph of L-shaped antenna unit b—3-D map of L-shaped antenna unit c—the scattering amplitude of single L-shaped antenna unit with the change of h and r d— the scattering phase of single L-shaped antenna unit with the change of h and r
![]()
Figure 2. a—the overlay phase array: array A(2π) and array B(2×2π) b—the normalized amplitude and phase diagram of the polarized light vertically
![]()
Figure 3. a—the 1st order transmission spectrum parallel to the polarization direction of incident light b—the 1st order transmission spectrum perpendicular to the polarization direction of incident light c— the 2nd order transmission spectrum parallel to the polarization direction of incident light d—the 2nd order transmission spectrum perpendicular to the polarization direction of incident light
![]()
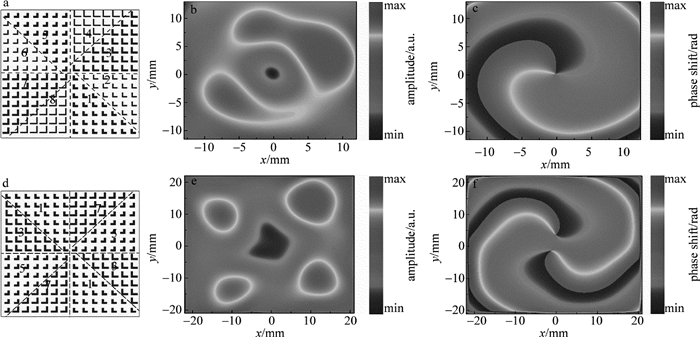
Figure 4. a—vortex phase plate under topological charge l=1 b—the normalized amplitude under topological charge l=1 c—the vortex phase under topological charge l=1 d—vortex phase plate under topological charge l=2 e—the normalized amplitude under topological charge l=2 f—the vortex phase under topological charge l=2
-
[1] WANG B, ZHANG Y. Design and application of THz metamaterials and matesurfaces[J].Journal of Terahertz Science and Electronic Information Technology, 2015, 13(1):1-12(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTotal-XXYD201501002.htm
[2] CHEN H T, TAYLOR A J, YU N F. A review of metasurfaces:physics and applications[J]. Report on Progress in Physical Society, 2016, 79(7):076401. DOI: 10.1088/0034-4885/79/7/076401
[3] HOLLOWAY C L, DIENSTFREY A, KUESTER E F, et al. A discussion on the interpretation and characterization of metafilms/metasurfaces:The two-dimensional equivalent of metamaterials[J]. Metamaterials, 2009, 3(2):100-112. http://www.sciencedirect.com/science/article/pii/S1873198809000231
[4] SKALAEV M I, SUN J B, TSUKERNIK A, et al. High-efficiency all-dielectric metasurfaces for ultracompact beam manipulation in transmission mode[J]. Nano Letters, 2015, 15(9):6261-6266. DOI: 10.1021/acs.nanolett.5b02926
[5] YU N F, GENEVET P, KATS M A, et al. Light propagation with phase discontinuities:generalized laws of reflection and refraction[J]. Science, 2011, 334(6054):333-337. DOI: 10.1126/science.1210713
[6] QIN F, DING L, ZHANG L, et al. Hybrid bilayer plasmonic metasurface efficiently manipulates visible light[J]. Science Advances, 2016, 2(1):1501168. http://pubmedcentralcanada.ca/pmcc/articles/PMC4705036/
[7] MO W C, WEI X L, WANG K J, et al. Ultrathin flexible terahertz polarization converter based on metasurfaces[J]. Optics Express, 2016, 24(12):13622-13627. http://europepmc.org/abstract/med/27410377
[8] HU D, WANG X K, FENG S F, et al. Ultrathin terahertz planar elements[J]. Advanced Optical Materials, 2013, 1(2):186-191. DOI: 10.1002/adom.201200044/pdf
[9] GENEVET P, YU N F, AIETA F, et al. Ultra-thin plasmonic optical vortex plate based on phase discontinuities[J]. Applied Physics Le-tters, 2012, 100(1):1-11. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=81bc74a41e54f60d775a9cf27a96cb75
[10] HE J W, WANG X K, HU D, et al. Generation and evolution of the terahertz vortex beam[J]. Optics Express, 2013, 21(17):20230-20239. DOI: 10.1364/OE.21.020230
[11] YANG Y M, WANG W Y, MOITRA P, et al. Dielectric meta-reflectarray for broadband linear polarization conversion and optical vortex generation[J]. Nano Letters, 2014, 14(3):1394-1399. DOI: 10.1021/nl4044482
[12] ALLEN L, BEIJERSBERGEN M W, SPREEUW R J C, et al. Orbital angular momentum of light and transformation of Laguerre Gaussian laser modes[J]. Physical Review, 1992, A45(11):8185-8189. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=CC0210219387
[13] BLACK L J, WANG Y D, GROOT C H, et al. Optimal polarization conversion in coupled dimer plasmonic nanoantennas for metasurfaces[J]. Acs Nano, 2014, 8(6):6390-6399. DOI: 10.1021/nn501889s
[14] WANG W, GUO Z Y, LI R Z, et al. Plasmonics metalens independent from the incident polarizations[J]. Optics Express, 2015, 23(13):16782-16791. DOI: 10.1364/OE.23.016782
[15] YU N F, GENEVET P, AIETA F, et al. Flat optics:Controlling wavefronts with optical antenna metasurfaces[J]. IEEE Journal of Selected Topics in Quantum Electronics, 2013, 19(3):4700423. DOI: 10.1109/JSTQE.2013.2241399
[16] WEI Y, ZHU Y Y. Analysis of phase change of Laguerre-Gaussian vortex beam during propagation[J]. Laser Technology, 2015, 39(5):723-726(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgjs201505029
[17] ZHANG Y X, XU J C, SI C F, et al. Effect of turbulent tilt, coma and astigmatism on orbital angular momentum state of laser beam[J]. Laser Technology, 2010, 34(6):747-749(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgjs201006008
[18] MAIR A, VAZIRI A, WEIHS G, et al. Entanglement of the orbital angular momentum states of photons[J]. Nature, 2001, 412(6844):313-316. DOI: 10.1038/35085529
下载:
计量
- 文章访问数: 5
- HTML全文浏览量: 2
- PDF下载量: 7