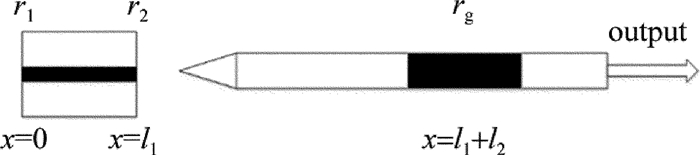
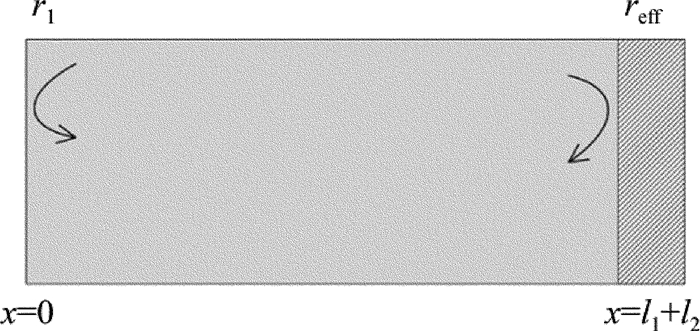
Selection and analysis of theoretical model of fiber Bragg grating external cavity laser diode
-
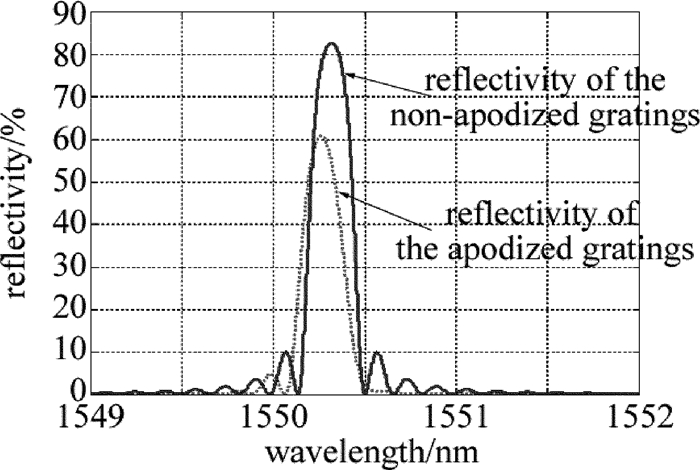
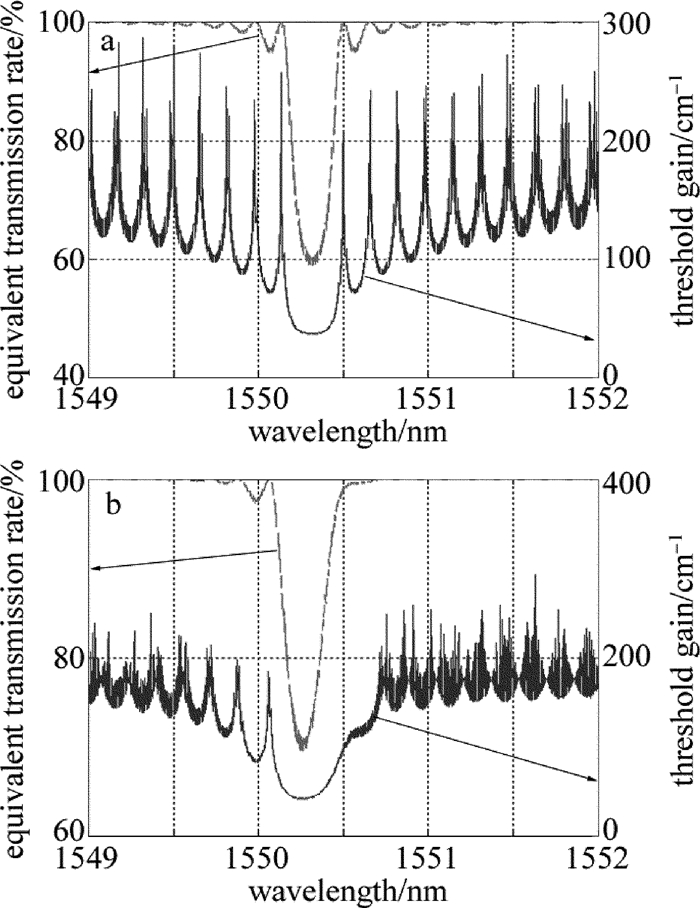
摘要: 为了讨论三腔镜近似或耦合腔近似两种等效腔模型对计算光纤光栅外腔半导体激光器等效反射率等参量的近似程度，挑选出最优的理论模型，采用数值计算的方法进行模拟仿真，得到了两种模型在采用切趾光纤Bragg光栅和非切趾光纤Bragg光栅情况下的阈值增益等特性曲线。结果表明，在采用切趾光纤Bragg光栅，特别是切趾深度较大的情况下，采用三腔镜近似模型更加符合实际情况。此研究结果对分析和设计单纵模光纤光栅外腔半导体激光器具有一定的参考价值。Abstract: In order to discuss approximation degree of calculating equivalent reflectivity and other parameters of the fiber Bragg grating external cavity laser diode by using two equivalent cavity models of the three cavity mirrors approximation or the coupled cavity approximation and choose the best one, the threshold gain characteristic curves of two approximation models by using apodized fiber Bragg grating and normal fiber Bragg grating were gotten after numerical caculation and simulation. Numerical analysis results show that three cavity mirrors approximate model is more in line with the actual situation when using the apodized fiber Bragg grating. This conclusion has the reference in analysing and designing single longitudinal mode fiber grating external cavity semiconductor laser.
-
-
![]()
Figure 1. Refractive index profile of fiber Bragg grating before and after apodization
![]()
Figure 8. Equivalent reflectivity of two models when using non-apodized grating
a—the three cavity mirrors model when using fiber Bragg gratings before apodized b—the coupled cavity model when using fiber Bragg gratings before apodized
![]()
Figure 9. Equivalent reflectivity of two models when G=5
a—the three cavity mirrors model b—the coupled cavity model
![]()
Figure 10. Equivalent reflectivity of two models when G=200
a—the three cavity mirrors model b—the coupled cavity model
![]()
Figure 11. Equivalent transmission and gain threshold when using the apodized and non-apodized gratings
a—the equivalent transmission and gain threshold when using fiber Bragg gratings before apodized b—the equivalent transmission and gain threshold when using Gaussian-apodized fiber Bragg gratings
Table 1 Calculation date
parameter value original refractive index of optical fiber core neff 1.46 fringe visibility m 0.5 length of the fiber lg 5mm average refractive index change \overline {{\rm{ \mathsf{ δ} }}{\mathit{n}_{{\rm{eff}}}}} 0.0003 length of the external cavity l2 10mm reflectivity coefficient of the gain chip's back-end r1 \sqrt {0.9} reflectivity coefficient of the gain chip's front-end r2 0.01 reflectivity of fiber facet r3 0.05 coupling coefficient η 70% refractive index of the semiconductor chip n1 3.4 length of the active region of semiconductor l1 300μm cavity loss α 20cm-1 extinction coefficient of air ka 0.01 apodization degree G 5 
下载: 导出CSV
-
[1] ALALUSI M, BRASIL P, LEE S, et al. Low noise planar external cavity laser for interferometric fiber optic sensors[J].Proceedings of the SPIE, 2009, 7316:433-443. http://spie.org/x648.xml?product_id=828849
[2] HU Z Y, ZHANG Y S, MA X Y, et al. Study of the characteristics of laser diode with fiber Bragg grating external cavity[J]. Chinese Journal of Lasers, 2000, 27(8):677-681(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zgjg200008002
[3] WANG L L, REN J H, ZHAO T G, et al. Study for realizing wavelength conversion of fiber grating external cavity semiconductor laser[J]. Opto-Electronic Engineering, 2005, 32(2):5-8(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gdgc200502002
[4] PAN B W, YU L Q, LU D, et al. 20kHz narrow linewidth fiber Bragg grating external cavity semiconductor laser[J]. Chinese Journal of Lasers, 2015, 42(5):0502007(in Chinese). DOI: 10.3788/CJL
[5] NUMATA K, ALALUSI M, STOLPNER L, et al. Characteristics of the single-longitudinal-mode planar-waveguide external cavity diode laser at 1064nm[J]. Optics Letters, 2014, 39(7):2101-2104. DOI: 10.1364/OL.39.002101
[6] NUMATA K, CAMP J, KRAINAK M, et al. Performance of planar-waveguide external cavity laser for precision measurements[J]. Optics Express, 2010, 18(22):22781-22788. DOI: 10.1364/OE.18.022781
[7] WANG L L, REN J H, ZHAO T G, et al. Theoretical and experimental study on a fiber grating external cavity semiconductor laser[J]. Laser Technology, 2005, 29(4):361-363(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgjs200504010
[8] ZHOU H Q, XIA G Q, DENG T, et al. Influence of external cavity length variation on the lasing wavelength of the fiber grating external cavity semiconductor laser[J]. Laser Technology, 2005, 29(5):476-477(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=8c3a1d695bac3231917b854859738a28
[9] CHEN Sh J, LI Y, YUAN W R, et al. Improvement of the spectral characteristics of 980nm semiconductor laser[J]. Acta Photonica Sinica, 2015, 44(1):0114003(in Chinese). DOI: 10.3788/gzxb
[10] YUN B F. Theoretical and experimental study of fiber Bragg grating sensors[D]. Nanjing: Southeast University, 2006: 17-31(in Chinese).
[11] HUANG W, HAN Y S, HE S L. A study on optical aperdization function for a fiber Bragg grating[J]. Journal of Optoelectronics·Laser, 2002, 13(12):1247-1251(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-GDZJ200212010.htm
[12] OLSSON A, TANG C L. Coherent optical interference effects in external-cavity semiconductor lasers[J]. IEEE Journal of Quantum Electronics, 1981, 17(8):1320-1323. DOI: 10.1109/JQE.1981.1071272
[13] OSMUNDSENJ, GADE N.Influence of optical feedback on laser frequency spectrum and threshold conditions[J].IEEE Journal of Quantum Electronics, 1983, 19(3):165-169. http://ieeexplore.ieee.org/xpls/abs_all.jsp?arnumber=1071857
[14] ZHOU H Q, WU Z M, XIA G Q. Influence of the temperature variation on the lasing wavelength of the fiber grating external cavity semiconductor laser[J]. Laser Journal, 2003, 24(4):65-66(in Chinese). http://www.sciencedirect.com/science/article/pii/S0030402604702661
[15] HE Y P, XIA G Q, DENG T, et al. Investigation on the output spectrum of fiber grating external cavity semiconductor lasers using ray tracing method[J]. Laser Journal, 2007, 28(3):18-19(in Chiinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgzz200703009
[16] CHAI Y J, ZHANG H Y, ZHOU B K. Linewidth performance analysis of semiconductor lasers with strong feedback external cavity[J]. Chinese Journal of Semiconductors, 1995, 16(12):885-889. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199500163739
[17] XIA G Q, WU Z M, CHEN J G. Theoretical model of external cavity semiconductor lasers including the reflectivity distribution of fiber grating[J]. Chinese Journal of Lasers, 2002, 29(4):301-303(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zgjg200204004
[18] WANG Y. InP-based multi-section coupled-cavity laser using deeply etched trenches[D]. Hangzhou: Zhejiang University, 2013: 60-76(in Chinese).
[19] HU C X, WU Z M, DENG T, et al. Influence of air gap on the lasing wavelength of the fiber grating external cavity semiconductor laser[J]. Laser Technology, 2008, 32(2):177-179(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgjs200802017
[20] WU X, RAN Y, LIU W P, et al. Research on performance of FBG sidelobes suppression based on double exposure apodizing technology[J]. Instrument Technique and Sensor, 2010(5):69-70(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-YBJS201005026.htm
[21] ZHAO T G, GAO H P, REN J H. The influence of the optical fiber grating on FBG-ECL's static characteristics[J]. Optical Technique, 2007, 33(1):116-118(in Chinese). http://en.cnki.com.cn/article_en/cjfdtotal-gxjs200701031.htm
[22] HONG C S, XU X H, LÜ G C, et al. Technologies and new progress of apodized fiber gratings[J]. Optoelectronic Technology and Information, 2002, 15(3):1-6(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gdzjsyxx200203001
-
期刊类型引用(4)
1. 崔文超, 郭瑞民, 王德发, 董贺伟. 分布反馈激光器温度与电流控制研究. 激光技术. 2019(04): 1-5 . 
本站查看
2. 陈垚至. 混沌激光通信网络波分复用传输系统设计. 激光杂志. 2018(10): 96-101 . 百度学术
3. 吴艳玲, 唐穗欣. 激光传感器的机器人运动控制研究. 激光杂志. 2017(01): 127-130 . 百度学术
4. 苏文芝, 申玉霞. 光纤信道混沌激光通信故障的提取与识别. 激光杂志. 2017(01): 144-147 . 百度学术
其他类型引用(0)
计量
- 文章访问数: 2
- HTML全文浏览量: 0
- PDF下载量: 17
- 被引次数: 4