光电跟踪系统的模糊Ⅱ型控制技术研究

秦树旺; 毛耀; 包启亮

doi:10.7510/jgjs.issn.1001-3806.2021.02.004

光电跟踪系统的模糊Ⅱ型控制技术研究

秦树旺^1,2,3,
毛耀^1,2,3, ,,
包启亮^1,2,3

1.
中国科学院光束控制重点实验室，成都 610209
2.
中国科学院光电技术研究所，成都 610209
3.
中国科学院大学，北京 100049

基金项目:

国家自然科学基金资助项目 6173000085

国家自然科学基金青年科学基金资助项目 6190032209

详细信息

通讯作者:
毛耀, E-mail: maoyao@ioe.ac.cn

中图分类号: TP273
计量
- 文章访问数: 4
- HTML全文浏览量: 1
- PDF下载量: 7
出版历程
- 收稿日期: 2020-04-01
- 修回日期: 2020-04-27
- 发布日期: 2021-03-24

Research on fuzzy Ⅱ-order control method of photoelectric servo tracking systems

QIN Shuwang^1,2,3,
MAO Yao^1,2,3, ,,
BAO Qiliang^1,2,3

1.
Key Laboratory of Optical Engineering, Chinese Academy of Sciences, Chengdu 610209, China
2.
Institute of Optics and Electronics, Chinese Academy of Sciences, Chengdu 610209, China
3.
University of Chinese Academy of Sciences, Beijing 100049, China

摘要

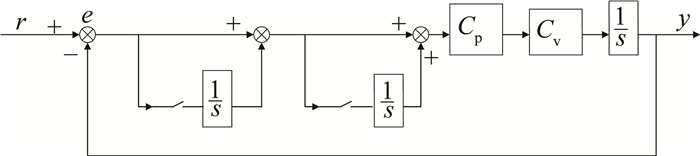
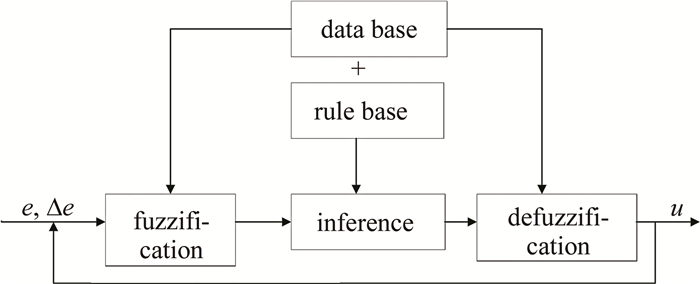
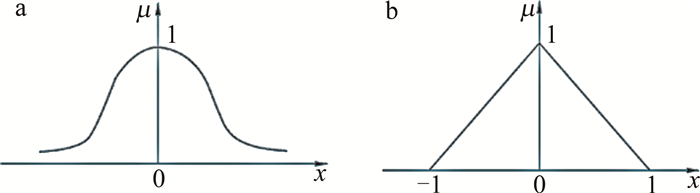
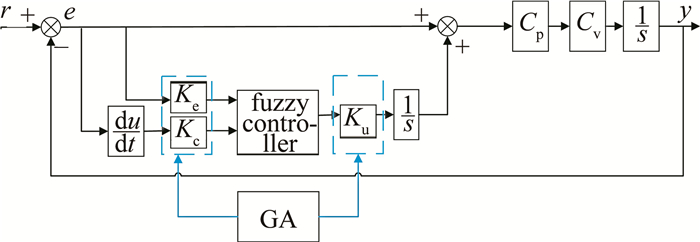
摘要: 为了解决高型控制方法在光电伺服跟踪系统应用中存在的稳态精度与超调量之间的矛盾，设计了一种由多种群遗传算法(MPGA)优化的模糊Ⅱ型控制方法。该方法在经典Ⅰ型双闭环反馈控制器的速度环之前并联一个积分环节，将系统型别增加到Ⅱ型，以此加快反应速度、减小稳态误差；并引入模糊控制器(FLC)，根据系统状态动态调节积分环节的增益，实现动态高型控制，既抑制系统震荡，又保证稳态精度；然后用MPGA优化FLC的输入输出比例因子，得到最优控制参量。对该控制系统进行了理论分析，并将各阶段优化系统进行了对比实验。结果表明，在相同实验条件下，采用MPGA优化的模糊Ⅱ型控制系统实现了对系统的动态高型控制，既能保证原系统的超调量不变，又能将稳态误差减小88.55%，明显提高了控制系统的稳态精度。该研究对优化光电伺服跟踪系统是有帮助的。
- 激光技术 /
- 动态高型控制系统 /
- 模糊控制 /
- 遗传算法
Abstract: In order to solve the contradiction between the steady-state accuracy and overshoot in the application of the high-order control method in the photoelectric servo tracking systems, a fuzzy Ⅱ-order controller optimized by multiple population genetic algorithm (MPGA) was designed. In this method, an integrator was connected in parallel before the speed loop of the classic Ⅰ-type double closed-loop feedback controller to increase the system order to the Ⅱ, thereby speeding up the reaction speed and reducing the steady-state error. Therefore, a fuzzy logic controller (FLC) was introduced to dynamically adjust the gain of the integrator according to the state of the system to achieve dynamic high-order control, which not only suppresses system oscillation but also ensures steady-state accuracy. MPGA was used to optimize the input and output scale factors of FLC to obtain the optimal control parameters. The control system was analyzed theoretically, and the optimized system in each stage was compared with experiments. The results show that under the same experimental conditions, the MPGA-optimized fuzzy Ⅱ-order control system is used to realize the dynamic high-order control of the system, which can not only ensure that the overshoot of the original system remains the same, but also reduce the steady-state error by 88.55%. This study significantly improves the steady-state accuracy of the control system. The research is helpful to optimize the photoelectric servo tracking system.
- laser technique /
- dynamic high-order control system /
- fuzzy logic control /
- genetic algorith

HTML全文

Figure 1. Double closed-loop control

下载: 全尺寸图片幻灯片

Figure 2. Dynamic high-order structure

下载: 全尺寸图片幻灯片

Figure 3. Classic FLC structure

下载: 全尺寸图片幻灯片

Figure 4. Membership function type

下载: 全尺寸图片幻灯片

Figure 5. Fuzzy-Ⅱ control system

下载: 全尺寸图片幻灯片

Figure 6. Membership function

a—input e b—input Δe c—input k

下载: 全尺寸图片幻灯片

Figure 7. Rule base 3-D distribution

下载: 全尺寸图片幻灯片

Figure 8. MPGA running process

下载: 全尺寸图片幻灯片

Figure 9. Step response comparison of step (1), (2), (3)

下载: 全尺寸图片幻灯片

Figure 10. Step response comparison of step (3), (4)

下载: 全尺寸图片幻灯片

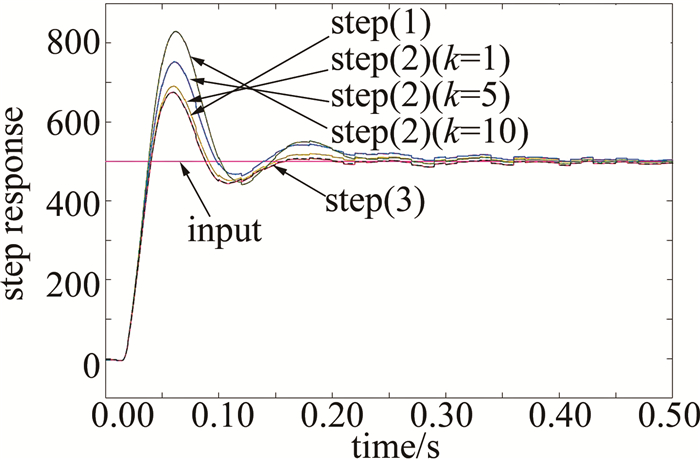
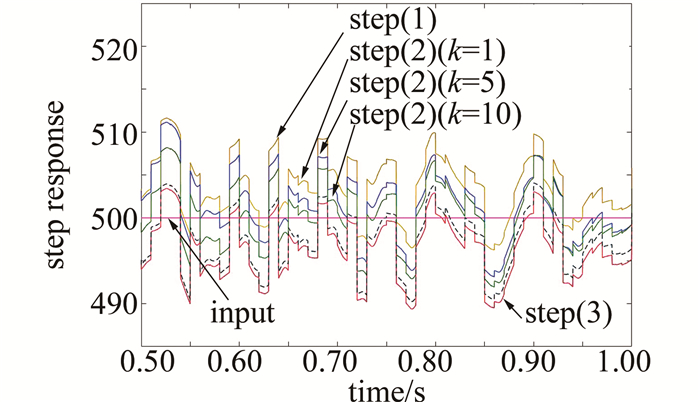
Figure 11. Enlarged steady-state view of 0.5s~1s in Fig. 9

下载: 全尺寸图片幻灯片

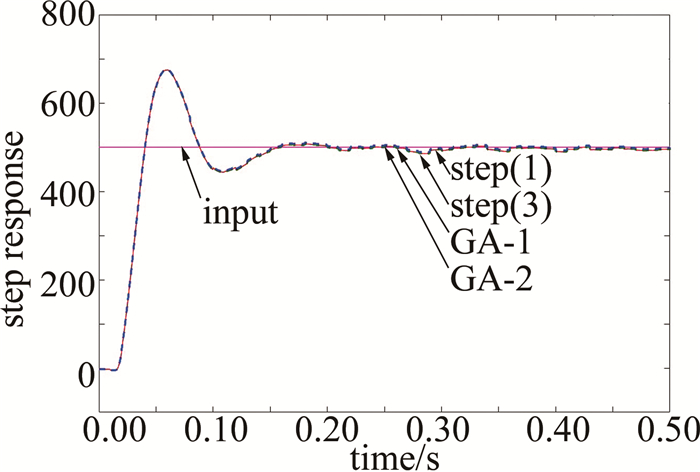
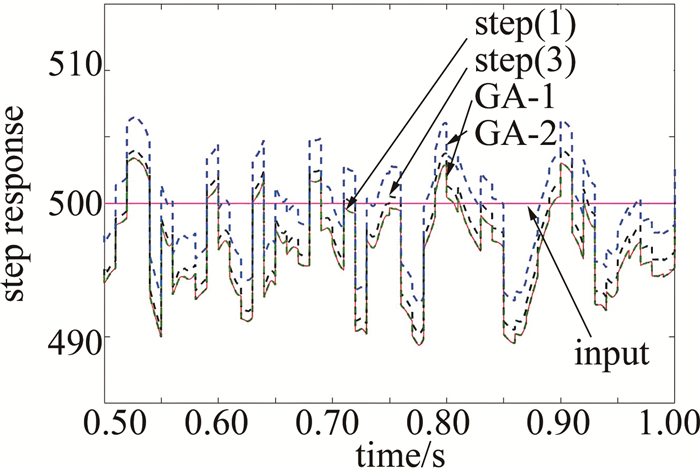
Figure 12. Enlarged steady-state view of 0.5s~1s in Fig. 10

下载: 全尺寸图片幻灯片

Figure 13. SGA evolution curve

下载: 全尺寸图片幻灯片

Figure 14. MPGA evolution curve

下载: 全尺寸图片幻灯片

Figure 15. Relationship between system error and FLC output

下载: 全尺寸图片幻灯片

Table 1 Fuzzy control rules

	N_b	N	Z	P	P_b
N_b	P_b	P_b	N	N_b	N_b
N	P_b	P	N_b	N	N
Z	N_b	N_b	N_b	N_b	N_b
P	N	N	N_b	Z	P
P_b	N_b	N_b	P	P_b	P_b

下载: 导出CSV

Table 2 Comparison of simulation results

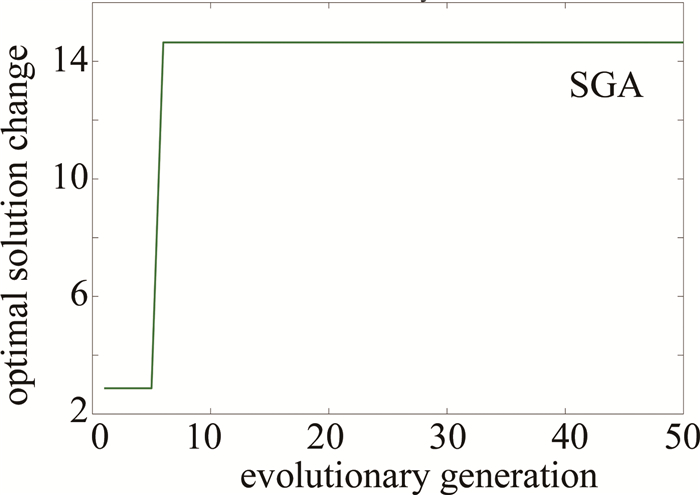
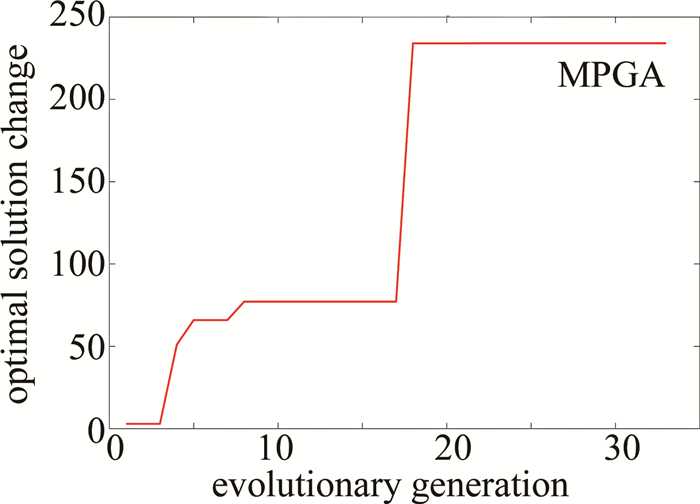
step		scale factor			J_ITAE	\|e_s\|
1		—			2.888	3.58
2			1		3.060	3.75
			k	5	3.400	1.62
			10		3.172	0.12
3		k_e	k_c	k_u	2.357	1.45
3		1	1	1	2.357	1.45
4	GA-1	0.0006	0.9994	0.001	2.873	3.56
4	GA-2	0.6953	0.3047	2.6288	2.238	0.41

下载: 导出CSV

参考文献(22)

[1]	XU Ch, HUANG D Q, LIU J Y. Target location of unmanned aerial vehicles based on the electro-optical stabilization and tracking platform[J]. Measurement, 2019, 147(7): 76-79. http://www.sciencedirect.com/science/article/pii/S0263224119307055
[2]	ZHAO T, CHEN Y, DIAN S, et al. General type-2 fuzzy gain sche-duling PID controller with application to power-line inspection robots[J]. International Journal of Fuzzy Systems, 2020, 22(9): 181-200. DOI: 10.1007/s40815-019-00780-1
[3]	HU Sh S. The principle of automatic control[M]. 5th ed. Beijing: Science Press, 2007: 471-472(in Chinese).
[4]	LIU Y, XIA R Q, LV Y. Research on composite PID controller used in fine pointer of beam[J]. Laser Technology, 2018, 42(6): 868-872 (in Chinese). http://en.cnki.com.cn/Article_en/CJFDTotal-JGJS201806027.htm
[5]	HORIUCHI T, INOUE M, KONNO T, et al. Real-time hybrid expe-rimental system with actuator delay compensation and its application to a piping system with energy absorber[J]. Earthquake Engineering & Structural Dynamics, 1999, 28(10): 1121-1141. DOI: 10.1002/(SICI)1096-9845(199910)28:10%3C1121:: AID-EQE858%3E3.0.CO; 2-O/abstract
[6]	MORARI M, LEE J H. Model predictive control: Past, present, and future[J]. Computers & Chemical Engineering, 1999, 23(4/5): 667-682. http://www.sciencedirect.com/science/article/pii/S0098135498003019
[7]	CAO Y, STUART D, REN W, et al. Distributed containment control for multiple autonomous vehicles with double-integrator dynamics: Algorithms and experiments[J]. IEEE Transactions on Control Systems Technology, 2010, 19(4): 929-938.
[8]	BRADLEY L M, CORRIVEAU J P, TINDAL N E. Launch area th-eodolite system[C]//Acquisition, Tracking, and Pointing: International Society for Optics and Photonics. New York, USA: IEEE, 1991: 48-60.
[9]	WANG J L, CHEN T, CHEN J, et al. A method for improving the tracking ability of a photoelectric theodolite against the fast moving targets[J]. Opto-Electronic Engineering, 2002, 29(1): 34-37(in Ch-inese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-GDGC200201009.htm
[10]	GENG A H, CHEN H, CHEN J, et al. Dynamic integral control for a digital servo system[J]. Journal of Changchun University of Technology (Natural Science Edition), 2007, 28(3): 261-263(in Ch-inese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-JLGX200703004.htm
[11]	SUN J. Study on application of dynamic high type method in tracking servo system of theodolite[D]. Changchun: Changchun Institute of Optics, Fine Mechanics and Physics, Chinese Academy of Science, 2004: 37-54(in Chinese).
[12]	LIU H, ZENG X H. Application of fuzzy-dynamic height control method in strapdown mirror stabilization platform[J]. Optics & Optoelectronic Technology, 2018, 16(2): 7-13(in Chinese).
[13]	LIU Sh T, CAO Y, PENG X F, et al. PID control of optical axis stabilization for airborne laser communication based on fuzzy neural network[J]. Laser Technology, 2017, 41(4): 606-610(in Chin-ese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-JGJS201704030.htm
[14]	QI Y Ch, CHEN W, MU Ch Y, et al. Laser temperature control system based on particle swarm self-tuning PID algorithm[J]. Laser Technology, 2019, 43(5): 650-654(in Chinese).
[15]	YANG Y, HUANG Zh Q, LIU F Y, et al. Application of multi-po-pulation genetic algorithm in wet steam parameter measurement[J]. Laser Technology, 2014, 38(4): 484-487(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-JGJS201404011.htm
[16]	LI J J, LIU D M, CHEN J. High-degree-mode dynamic control for theodolite tracking servo system[J]. Information and Control, 2006, 35(5): 647-650. http://en.cnki.com.cn/Article_en/CJFDTOTAL-XXYK200605019.htm
[17]	SHEN Y Ch, TANG W W, ZHANG G X, et al. Research of routing problem based on genetic algorithm[J]. Laser Technology, 2011, 35(3): 422-424(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-JGJS201103034.htm
[18]	ZHANG T F, ZHANG H X, MENG F, et al. Application of improved Hausdorff distance and quantum genetic algorithm in laser i-mage guidance[J]. Laser Technology, 2016, 40(3): 320-325(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-JGJS201603004.htm
[19]	WANG A X, FENG J. Genetic simulated annealing algorithin in the parameter retrieval of light scattering model[J]. Laser Technology, 2009, 33(1): 32-35(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTotal-JGJS200901011.htm
[20]	LIU M, WANG J H. Genetic algorithm optimized fuzzy controller method[J]. Journal of Fujian Computer, 2005(8): 40-41(in Ch-inese).
[21]	SUN J P, YANG Zh Y, YU X N, et al. Design of a fuzzy controller based on multilevel GAs[J]. Journal of North China Electric Power University, 2002, 29(4): 41-43(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-HBDL200204011.htm
[22]	XIAO C Sh, MAO Y H, YUAN H W. Design and simulation of intelligent control algorithm for quadrotors under wind disturbance[J]. Computer Science, 2018, 45(5): 317323(in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-JSJA201805056.htm

施引文献

资源附件(0)

图(15) / 表(2)

计量

文章访问数: 4
HTML全文浏览量: 1
PDF下载量: 7
被引次数: 0

光电跟踪系统的模糊Ⅱ型控制技术研究

通讯作者:
毛耀, E-mail: maoyao@ioe.ac.cn

计量

Research on fuzzy Ⅱ-order control method of photoelectric servo tracking systems

计量

目录

友情链接：

	N_b	N	Z	P	P_b
N_b	P_b	P_b	N	N_b	N_b
N	P_b	P	N_b	N	N
Z	N_b	N_b	N_b	N_b	N_b
P	N	N	N_b	Z	P
P_b	N_b	N_b	P	P_b	P_b

	N_b	N	Z	P	P_b
N_b	P_b	P_b	N	N_b	N_b
N	P_b	P	N_b	N	N
Z	N_b	N_b	N_b	N_b	N_b
P	N	N	N_b	Z	P
P_b	N_b	N_b	P	P_b	P_b

光电跟踪系统的模糊Ⅱ型控制技术研究

通讯作者: 毛耀, E-mail: maoyao@ioe.ac.cn

计量

出版历程

Research on fuzzy Ⅱ-order control method of photoelectric servo tracking systems

计量

出版历程

目录

友情链接：

通讯作者:
毛耀, E-mail: maoyao@ioe.ac.cn

	N_b	N	Z	P	P_b
N_b	P_b	P_b	N	N_b	N_b
N	P_b	P	N_b	N	N
Z	N_b	N_b	N_b	N_b	N_b
P	N	N	N_b	Z	P
P_b	N_b	N_b	P	P_b	P_b