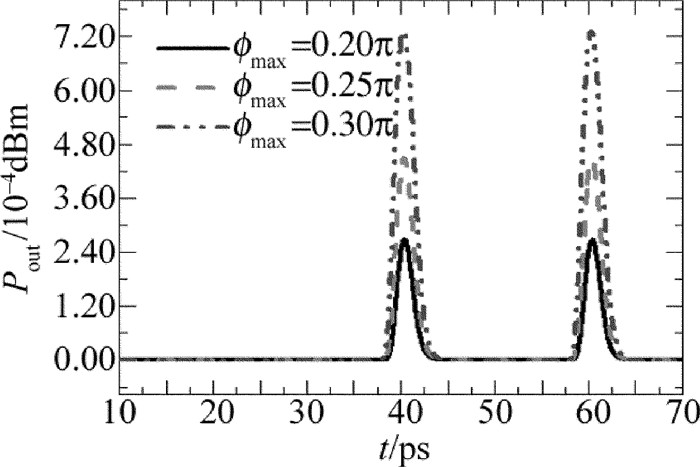
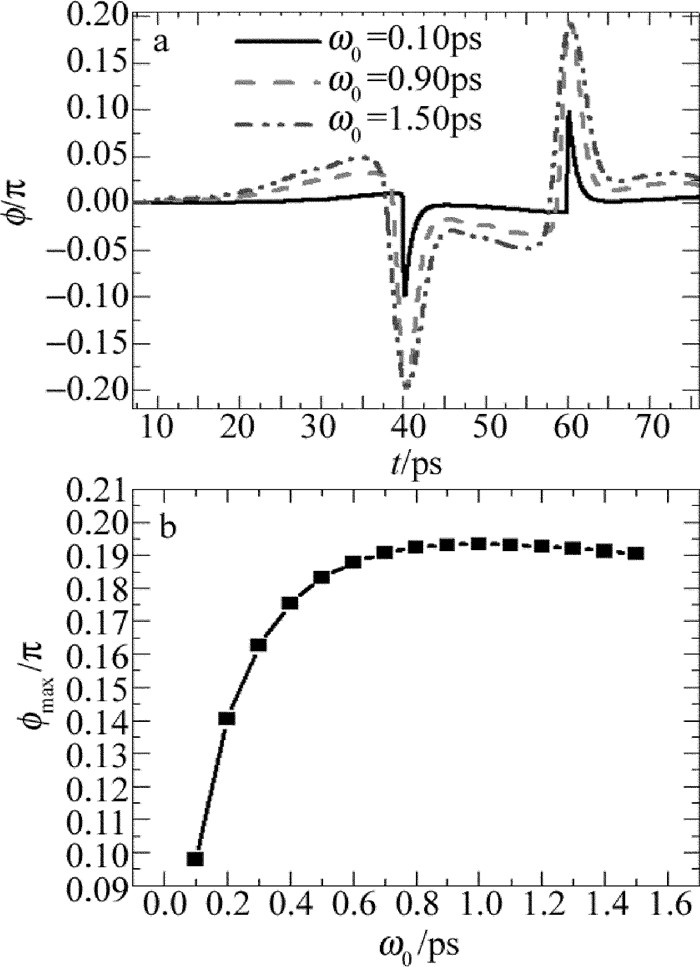
Study on phase difference of all-optical logic XOR gates
-
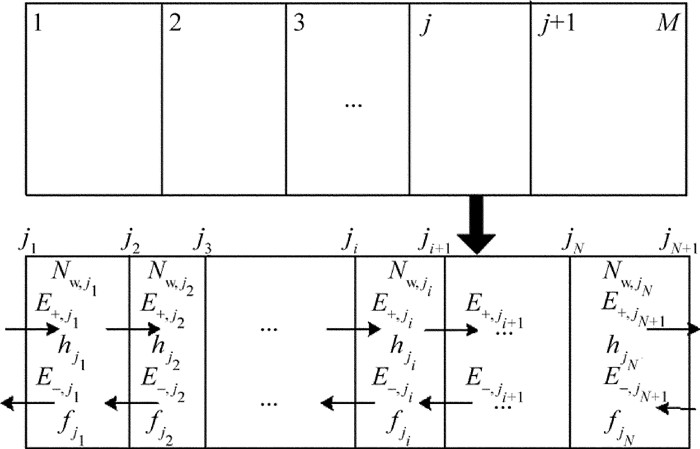
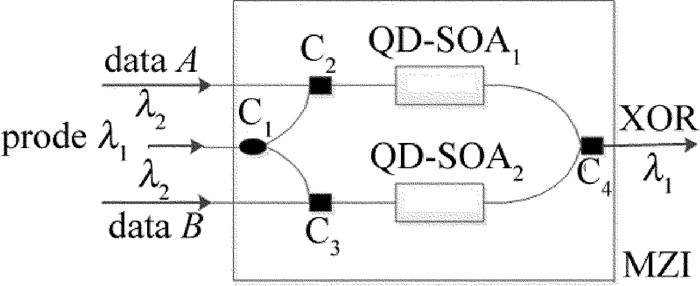
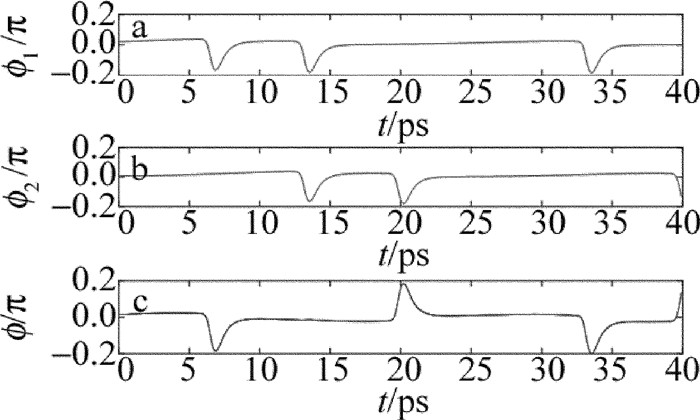
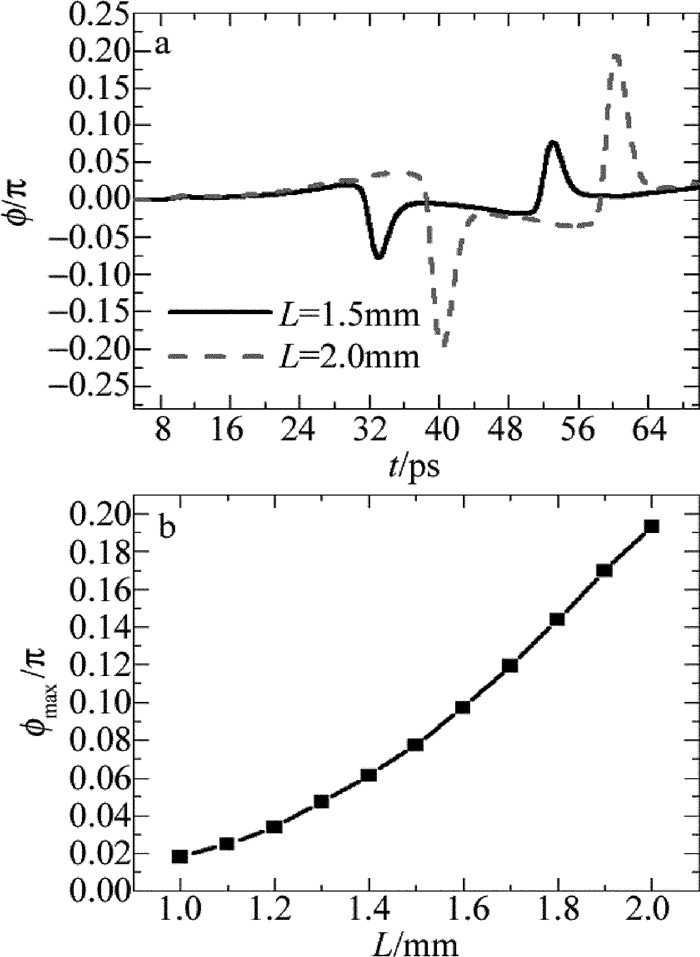
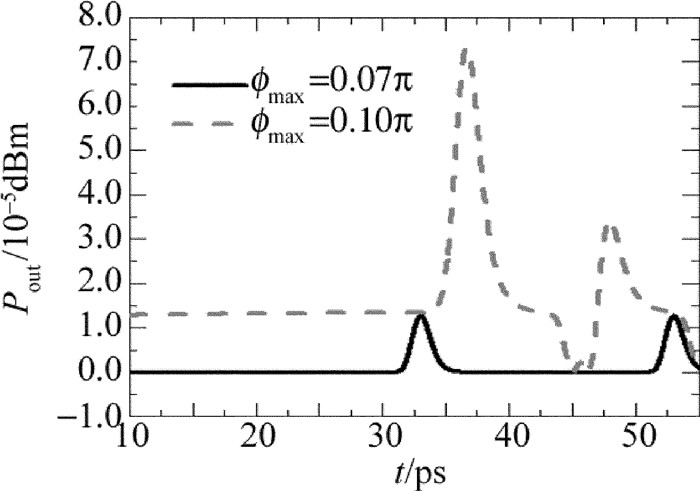
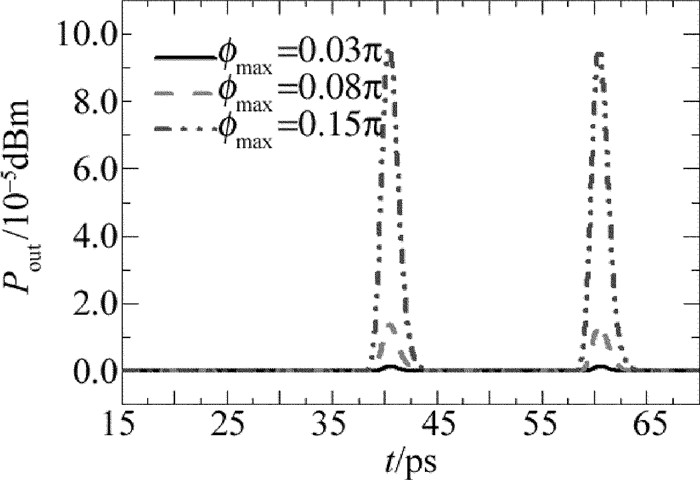
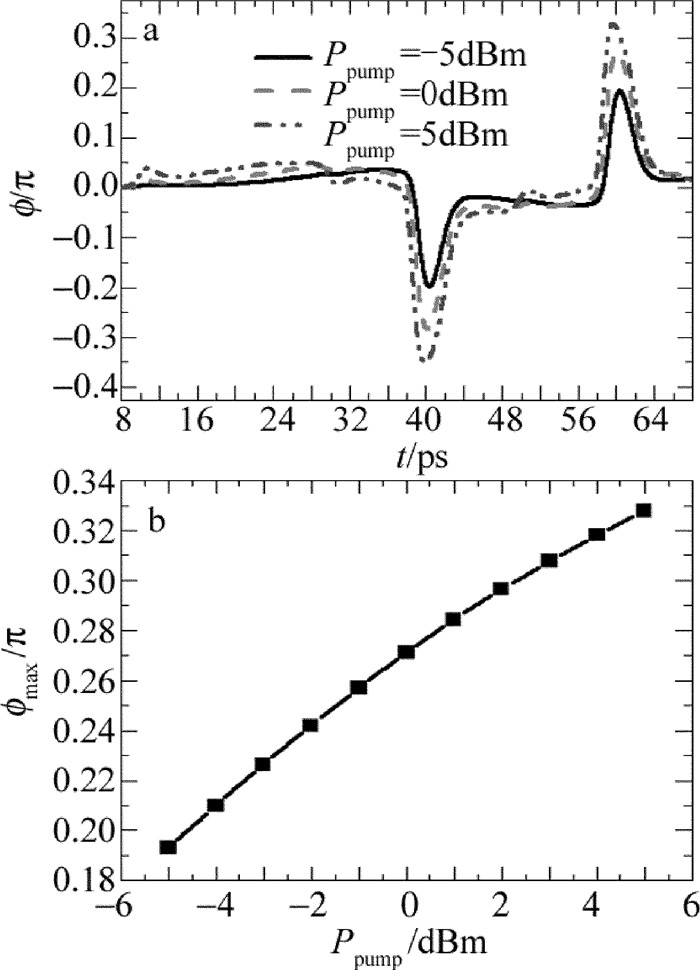
摘要: 为了改善全光逻辑门的相位差特性，对全光逻辑异或门的相位差进行了研究。采用细化分段模型对量子点半导体光放大器的动态过程进行建模，利用牛顿法和4阶龙格-库塔法求解三能级跃迁速率方程以及光场传输方程，实现了基于量子点半导体光放大器马赫-曾德尔干涉仪结构的全光逻辑异或门；研究了有源区长度、最大模式增益、输入抽运光功率以及输入抽运光脉冲宽度对通过干涉仪两臂探测光相位差的影响，同时讨论了探测光的相位差与输出光功率的关系。结果表明，增大有源区长度、最大模式增益以及输入抽运光功率，均能使探测光相位差增大；随着抽运光脉冲宽度增大，探测光相位差先增大而后趋于平缓，之后不断减小；有源区长度为2.0mm、最大模式增益为3000m-1、输入抽运光功率为5dBm、抽运光脉冲宽度为1.0ps时，最大相位差增加至0.3277π；随着探测光相位差增大，输出光功率增大；通过优化参量可以增大探测光的相位差，而输出光功率会随着探测光相位差的增大而增大。该研究为提高转换信号质量提供了参考。
-
关键词:
- 光通信 /
- 量子点半导体光放大器 /
- 异或门 /
- 相位差 /
- 输出光功率
Abstract: In order to optimize the phase difference of the all-optical logic gates, the phase difference of all-optical logic exclusive OR (XOR) gates was studied. Firstly, the refined sectionalized model was used simulate the dynamic process of quantum-dot semiconductor optical amplifier (QD-SOA). Secondly, the Newton method and the four-order Runge-Kutta method were used to solve the three-level transition rate equations and the light field transfer equations. Finally, an all-optical logic XOR gate based on quantum-dot semiconductor optical amplifier Mach-Zehnder interferometer (QD-SOA-MZI) was implemented. The influence of the length of the active regions, the maximum modal gain, input pump power and input pump pulse width on phase difference of probe signal through two arms of the interferometer was studied in detail. Moreover, the relationship between phase difference of the probe signal and output optical power was also discussed. The results show that, with the increase of the length of the active regions, the maximum modal gain and input pump power can lead to improve phase difference of probe signal through two arms of the interferometer. With the increase of input pump pulse width, phase difference of probe signal through two arms of the interferometer increases at first and then decreases. When the length of the active region is 2.0mm, the maximum modal gain is 3000m-1, the input pump power is 5dBm, and input pump pulse width is 1.0ps, the maximum phase difference of probe signal through two arms of the interferometer increases 0.3277π. Output optical power also can be improved by the increase of probe signal phase difference. Phase difference of the detected light can be increased by optimizing the parameters. Output light power increases with the increase of the phase difference of the probe. This study provides a reference for improving the quality of conversion signals. -
引言
随机共振现象是噪声和非线性动力学相互作用出现的一种有趣的现象。1981年被BENZI等人首次发现，此后在很多领域成为人们广泛研究的课题，在实验和理论研究上都取得了很大的进展。但是现有的研究成果大多受限于单一频率的周期驱动信号。而在实践应用中，需要使载波振幅按照调制信号的改变而实行调制，使其保持着高频载波的频率特性, 需要将此调制信号加载到激光源上，使其作为传递信息的工具。这对激光通讯和提高激光的效率有重要的作用。通常，传统的随机共振一般由信噪比与噪声强度的关系来体现。1998年，BARZYKIN等人提出可以用信噪比与噪声自相关时间的关系来体现。CHENG等人用信噪比与净增益系数之间的变化关系也阐明了这点。后来的研究说明了随机共振可由信噪比与系统中其它参量之间的关系来体现。作者用线性化近似方法计算了在偏置调幅信号下，色抽运噪声和实部、虚部间关联的量子噪声驱动的单模激光损失模型的输出光强信噪比，发现信噪比与系统的净增益系数之间存在共振现象。此外，还讨论了输入信号和噪声在量子噪声实部虚部间弱关联和强关联时对随机共振的影响，为优化激光系统的动力学性质提供依据。
1. 输入偏置调幅波的单模激光损失模型的光强关联函数及信噪比
单模激光损失模型输入偏置调幅波后的光强方程为：
dIdt′=2a0I−2AI2+Q (1−|λ|)+2Ipr(t′)+2√Iεr(t′)+B[1−Dcos(Ωt′)]cos(ωt′) (1) 抽运噪声p(t′)和量子噪声ε(t′)的统计性质为:
{⟨pr(t′)⟩=⟨εr(t′)⟩=0⟨pr (t) pr(t′)⟩=P2τexp(|t−t′|τ)⟨εr(t)εr(t′)⟩=Q (1+|λ|)δ(t−t′)⟨pr(t)εe(t′)⟩=⟨pr(t′)εr(t)⟩=0 (2) 式中，a0为净增益系数, A为自饱和系数; I为光强, B为载波信号振幅，Ω为低频调制信号频率；pr(t′)为抽运噪声实部, εr(t′)为位相锁定后的量子噪声；P和Q是抽运噪声强度和量子噪声强度, τ为抽运噪声自关联时间, ω为高频载波信号频率, D为调制信号振幅；λ为量子噪声实部、虚部之间的关联系数，其取值范围为λ≤1。本文中所讨论的物理量，均无量纲。
设I=I0+δ(t′)，δ(t′)为微小扰动项，I0为定态光强。在I0=a0/A附近, 对(1)式线性化处理得：
dδ(t′)/dt′=−γδ(t′)+Q (1−|λ|)+2I0pr(t′)+2√I0εr(t′)+B[1−Dcos(Ωt′)]cos(ωt′) (3) 式中, γ=2a0。
定义归一化稳态平均光强关联函数：
C(t)=lim (4) 进行傅里叶变换, 得出光强的功率谱：
S\text{ }\left( \omega ' \right)={{S}_{1}}\left( \omega ' \right)+{{S}_{2}}\left( \omega ' \right) 式中, S1(ω′)为输出信号功率谱, S2(ω′)为输出噪声功率谱。输出信号功率谱中有3个信号频率，输出总信号功率为：
{{P}_{S}}=\int_{0}^{\infty }{{{S}_{1}}(\omega ')\text{ d}\omega '} (5) 信噪比的定义为输出信号功率与3个信号频率处单位噪声功率之和的比值(只取正ω的谱):
R=\frac{{{P}_{S}}}{{{S}_{2}}\left( \omega \right)+{{S}_{2}}\left( \omega +\mathit{\Omega } \right)+{{S}_{2}}(\omega -\mathit{\Omega })} (6) 式中,
\begin{matrix} {{P}_{S}}=\frac{{{\text{ }\!\!\pi\!\!\text{ }}^{2}}{{B}^{2}}{{D}^{2}}}{4{{I}_{0}}^{2}{{\left[ {{\gamma }^{2}}+{{\left( \mathit{\Omega }+\omega \right)}^{2}} \right]}^{2}}}\left[ \frac{{{\gamma }^{2}}}{\mathit{\Omega }+\omega }+\left( \mathit{\Omega }+\omega \right) \right]+ \\ \frac{{{\text{ }\!\!\pi\!\!\text{ }}^{2}}{{B}^{2}}{{D}^{2}}}{4{{I}_{0}}^{2}{{\left[ {{\gamma }^{2}}+{{\left( \mathit{\Omega }-\omega \right)}^{2}} \right]}^{2}}}\left[ \frac{{{\gamma }^{2}}}{\mathit{\Omega }-\omega }+\left( \mathit{\Omega }-\omega \right) \right]+\frac{{{\text{ }\!\!\pi\!\!\text{ }}^{2}}{{B}^{2}}}{{{I}_{0}}^{2}\Omega ({{\gamma }^{2}}+{{\omega }^{2}})} \\ \end{matrix} (7) \left\{ \begin{align} &{{S}_{2}}\left( \omega \right)=\frac{2\text{ }\!\!\pi\!\!\text{ }{{Q}^{2}}{{\left( 1-\left| \lambda \right| \right)}^{2}}}{{{I}_{0}}^{2}{{\gamma }^{2}}}+\frac{4P{{\tau }^{2}}}{({{\gamma }^{2}}{{\tau }^{2}}-1)({{\omega }^{2}}{{\tau }^{2}}+1)}+ \\ &\frac{4}{{{\gamma }^{2}}+{{\omega }^{2}}}\left[ \frac{Q\text{ }\left( 1+\left| \lambda \right| \right)}{{{I}_{0}}}-\frac{P}{{{\gamma }^{2}}{{\tau }^{2}}-1} \right] \\ &{{S}_{2}}\left( \omega +\mathit{\Omega } \right)=\frac{2\text{ }\!\!\pi\!\!\text{ }{{Q}^{2}}{{\left( 1-\left| \lambda \right| \right)}^{2}}}{{{I}_{0}}^{2}{{\gamma }^{2}}}+\frac{4P{{\tau }^{2}}}{({{\gamma }^{2}}{{\tau }^{2}}-1)\left[ {{\left( \omega +\mathit{\Omega } \right)}^{2}}{{\tau }^{2}}+1 \right]}+ \\ &\frac{4}{{{\gamma }^{2}}+{{\left( \omega +\mathit{\Omega } \right)}^{2}}}\left[ \frac{Q\left( 1+\left| \lambda \right| \right)}{{{I}_{0}}}-\frac{P}{{{\gamma }^{2}}{{\tau }^{2}}-1} \right] \\ &{{S}_{2}}\left( \omega -\mathit{\Omega } \right)=\frac{2\text{ }\!\!\pi\!\!\text{ }{{Q}^{2}}{{\left( 1-\left| \lambda \right| \right)}^{2}}}{{{I}_{0}}^{2}{{\gamma }^{2}}}+\frac{4P{{\tau }^{2}}}{({{\gamma }^{2}}{{\tau }^{2}}-1)\left[ {{\left( \omega -\mathit{\Omega } \right)}^{2}}{{\tau }^{2}}+1 \right]}+ \\ &\frac{4}{{{\gamma }^{2}}+{{\left( \omega -\mathit{\Omega } \right)}^{2}}}\left[ \frac{Q\text{ }\left( 1+\left| \lambda \right| \right)}{{{I}_{0}}}-\frac{P}{{{\gamma }^{2}}{{\tau }^{2}}-1} \right] \\ \end{align} \right. (8) 2. 单模激光系统的随机共振现象
由(6)式得出的信噪比R和净增益系数a0的关系曲线出现了一个极大值，也就是单峰随机共振现象。
2.1 偏置信号对随机共振的影响
图 1为(6)式中以偏置信号振幅B为参量画出的R-a0曲线。参量为A=1, P=0.001, Q=0.01, τ=0.02, Ω=0.01, ω=100, D=1.5。由于(1)式的导出利用了统一色噪声近似，故所取的抽运噪声色关联时间 \tau \ll 1 。在图 1a中可以看到，当λ=0.1(弱关联)时，信噪比R随a0的变化出现一个共振峰，且随着偏置信号振幅B的线性增加，R的峰值也几乎线性增加，说明输入的偏置信号振幅越强，随机共振越强。从图 1b可以看出，在λ=0.9(强关联)的情况下，曲线R-a0也出现一个共振峰，随着输入信号振幅B的线性增加，峰值也几乎线性增加，并且峰值比弱关联时更大，同时，共振峰变得更加尖锐，位置离阈值更近。表明在激光系统中量子噪声实部、虚部间关联强度越强，输入信号振幅越大，系统的随机共振越明显。
以低频调制信号频率Ω为参量时，由(6)式画出的R-a0曲线, 如图 2所示。参量为A=1, P=0.001, Q=0.01, τ=0.02, B=1, ω=100, D=1.5。由图 2a中可以看到，在λ=0.1(弱关联)时，信噪比R随a0的变化出现一个共振峰，且随Ω的线性减小，R的峰值出现非线性增加，表明低频调制信号频率越小，对随机共振影响越大。从图 2b可以看出，在λ=0.9(强关联)情况下，信噪比R随a0的变化也出现一个共振峰，且峰值较弱关联时增大，表明量子噪声实部、虚部间的关联越强，共振峰越尖锐，对随机共振影响越大。
以高频载波信号频率ω为参量时，由(6)式画出的R-a0曲线如图 3所示。参量为A=1, P=0.001, Q=0.01, τ=0.02, B=1, Ω=0.01, D=1.5。由图 3a中可以看到，在λ=0.1(弱关联)时，信噪比R随a0的变化出现一个共振峰，且当ω线性减小时，R的峰值出现非线性增加，峰值位置左移，表明高频载波信号频率稍小时，随机共振越强。从图 3b可以看出，在λ=0.9(强关联)情况下，信噪比R随a0的变化也出现一个共振峰，且峰值较弱关联时增大，表明量子噪声实虚部间的关联越强，共振峰越高，越尖锐，越接近阈值，对随机共振影响越大。
2.2 噪声对随机共振的影响
以抽运噪声强度P为参量时，由(6)式画出的R-a0曲线如图 4所示。参量为A=1, Q=0.01, τ=0.02, Ω=0.01, ω=100，B=1, D=1.5。由图 4a中可知，R随a0的变化出现峰值，当λ=0.1(弱关联)、且接近阈值时，曲线R-a0基本不随P的变化而变化；稍远离阈值时，峰值随着P的线性减小而几乎线性增加。当λ=0.9(强关联)时，如图 4b所示，曲线R-a0几乎不随P的变化而变化，但当抽运噪声强度相同时(P=0.001)，图 4b中比图 4a中出现的共振峰值要大，峰更尖锐。由图说明，量子噪声实部、虚部间强关联情况下，抽运噪声强度的变化对随机共振的影响很小；同时，量子噪声实部、虚部间的关联越强，随机共振现象越明显。
以量子噪声强度Q为参量，由(6)式画出R-a0的曲线如图 5所示。参量为A=1, P=0.001, τ=0.02, Ω=0.01, ω=100，B=1, D=1.5。由图 5a中可知，当λ=0.1(弱关联)时，曲线R-a0出现一个共振峰，当Q线性减小时，R的峰值出现非线性增加，且共振峰位置稍稍左移，表明量子噪声强度越小，随机共振越强。从图 5b可以看出，在λ=0.9(强关联)情况下，信噪比R随a0的变化也出现一个共振峰，当两图取相同的量子噪声强度时，量子噪声实部、虚部间的关联越强，随机共振峰越高，越尖锐，越接近阈值。图 5表明，量子噪声实部、虚部间关联越强，量子噪声强度越小，随机共振现象越明显。
3. 结论
(1) 当调制信号振幅增大、低频调制信号频率减小、高频载波信号频率较小以及量子噪声强度、抽运噪声强度均减小时，系统的随机共振加强。
(2) 量子噪声实部、虚部间关联越强，随机共振现象越明显，峰值越大。
(3) 强关联下(λ=0.9)，当激光系统的参量确定时，抽运噪声强度对随机共振现象几乎不影响。
(4) 利用类似的研究发现，输入偏置信号后，计算得出的信噪比与信号中高频载波信号频率也会出现共振峰，即随机共振现象。
参考文献[15]中研究了输入单一频率周期信号时信号和噪声对随机共振现象的影响，发现当量子噪声实部、虚部之间弱关联时(λ=0)出现较明显的共振峰，这与本文中研究的偏置信号输入弱关联时(λ=0)，随机共振峰小且不明显的研究情况刚好相反。因为参考文献[15]中得到的信噪比R只与(1+|λ|)这一项有关，但本文中, 而除此项外, 还与(1-|λ|)3有关。对于量子噪声强度和抽运噪声强度对随机共振的影响而言，两者结论一致。
-
![]()
Figure 5. Phase of probe signals through two arms of MZI
a—QD-SOA1 b—QD-SOA2 c—phase difference between two arms of MZI
![]()
Figure 6. a—relationship between phase difference and time with different lengths b—relationship between phase difference and length of the active regions
![]()
Figure 8. Relationship between maximum modal gain and phase difference
a—phase difference varying with time in different maximum modal gain b—phase difference varying with maximum modal gain
![]()
Figure 9. Relationship between output optical power and time with different phase differences: 0.03π, 0.08π, 0.15π
![]()
Figure 10. a—relationship between phase difference and time with different pump power b—relationship between phase difference and pump power
![]()
Figure 11. Relationship between output optical power and time with different phase differences: 0.20π, 0.25π, 0.30π
![]()
Figure 12. a—relationship between phase different and time b—relationship between with phase different and pulse width
![]()
Figure 13. Relationship between output optical power and time at different phase differences
Table 2 Parameters for numerical calculation
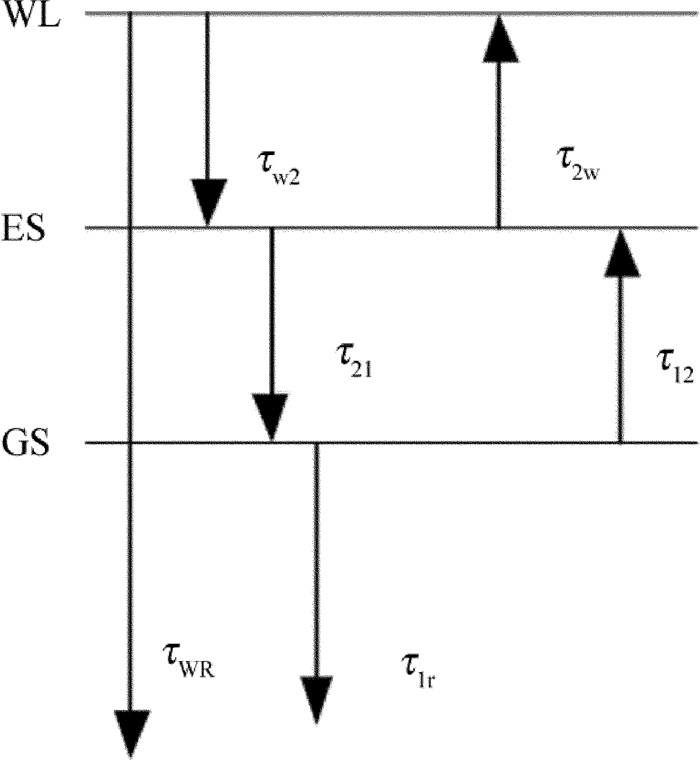
parameter value optical confinement factor Γ 0.5 absorption coefficient of the material αint 300m－1 thickness of wetting layer Lw 0.2μm surface density of QD Nq 5×1014m－2 spontaneous radiative lifetime in QD τ1r 0.4ns spontaneous radiative lifetime in WL τWR 2ns electron escape time from GS to ES τ12 1.2ps electron relaxation time from ES to GS τ21 0.16ps electron escape time from ES to WL τ2w 1ns electron relaxation time from WL to ES τw2 3ps 
下载: 导出CSV
-
[1] CHEN D, WANG R, PU T, et al. A novel thresholder based on XGM effect in a DFB laser combined with external optical filtering[J]. IEEE Photonics Journal, 2016, 8(1): 1-7. http://ieeexplore.ieee.org/document/7393448/
[2] SHI Sh Sh, WANG H L, GONG Q, et al. Refined sectionalized method of QD-SOA[J]. Optik-International Journal for Light and Electron Optics, 2014, 125(1): 504-507. http://www.sciencedirect.com/science/article/pii/S0030402613009649
[3] SINGH P, TRIPATHI D K, JAISWAL S, et al. Designs of all-optical buffer and OR gate using SOA-MZI[J]. Optical and Quantum Electronics, 2014, 46(11): 1435-1444. DOI: 10.1007/s11082-013-9856-0
[4] ZAJNULINA M, LINGNAU B, LVDGE K. Four-wave mixing in quantum-dot semiconductor optical amplifiers: A detailed analysis of the nonlinear effects[J]. IEEE Journal of Selected Topics in Quantum Electronics, 2017, 23(6): 1-12. http://ieeexplore.ieee.org/document/7876839/
[5] KOTB A. Simulation of high quality factor all-optical logic gates based on quantum-dot semiconductor optical amplifier at 1Tb/s[J]. Optik-International Journal for Light and Electron Optics, 2016, 127(1): 320-325. DOI: 10.1016/j.ijleo.2015.10.093
[6] CUI L L, WANG H L, LI W, et al. Study on gain recovery time of wavelength conversion based on single-port-coupled QD-SOA[J]. Laser Technology, 2016, 40(5): 742-745(in Chinese). http://www.jgjs.net.cn/EN/Y2016/V40/I5/742
[7] AMOR L. All-optical networks: Security issues analysis[J]. Journal of Optical Communications and Networking, 2015, 7(3): 136-145. DOI: 10.1364/JOCN.7.000136
[8] TAN H N, INOUE T, SOLIS-TRAPALA K, et al. On the cascadability of all-optical wavelength converter for high-order QAM formats[J]. Journal of Lightwave Technology, 2016, 34(13): 3194-3205. DOI: 10.1109/JLT.2016.2545246
[9] MATAUURA M, OHTA H, SEKI R. Experimental investigation of chirp properties induced by signal amplification in quantum-dot semiconductor optical amplifiers[J]. Optics Letters, 2015, 40(6): 914-917. DOI: 10.1364/OL.40.000914
[10] ALIMOHAMMADI F, YADIPOUR R, ABBASIAN K, et al. THz-assisted instantaneous gain switching in quantum dot semiconductor optical amplifiers[J]. IEEE Photonics Technology Letters, 2015, 27(3): 288-291. DOI: 10.1109/LPT.2014.2369514
[11] EYAL O, WILLINGER A, BANYOUDEH S, et al. Static and dynamic characteristics of an InAs/InP quantum-dot optical amplifier operating at high temperatures[J]. Optics Express, 2017, 25(22): 27262-27269. DOI: 10.1364/OE.25.027262
[12] YANG W H, WANG H L, WANG Zh X, et al.Wavelength conversion efficiency of quantum dot semiconductor optical amplifier[J].Acta Optica Sinica, 2017, 37(4):0406005(in Chinese). DOI: 10.3788/AOS
[13] CHEN T T, WANG H L, LIU S, et al. Theoretical study on chirp of wavelength conversion based on QD-SOA[J]. Laser Technology, 2016, 40(2): 292-295(in Chinese). http://www.opticsjournal.net/abstract.htm?id=OJ1603290001786B9EaH
[14] NURMOHAMMADI T, ABBASIAN K, AS'ADI M J, et al. Design of an ultrafast all-optical NOR logic gate based on Mach-Zehnder interferometer using quantum-dot SOA[J]. Optik-International Journal for Light and Electron Optics, 2014, 125(15): 4023-4029. DOI: 10.1016/j.ijleo.2014.01.119
[15] TALEB H, ABEDI K. Design of a low-power all-optical NOR gate using photonic crystal quantum-dot semiconductor optical amplifiers[J]. Optics Letters, 2014, 39(21): 6237-6240. DOI: 10.1364/OL.39.006237
[16] SINGH P, TRIPATHI D K, DIXIT H K. Designs of all-optical NOR gates using SOA based MZI[J]. Optik-International Journal for Light and Electron Optics, 2014, 125(16): 4437-4440. DOI: 10.1016/j.ijleo.2014.02.032
[17] GAYEN D K, CHATTOPADHYAY T. Designing of optimized all-optical half adder circuit using single quantum-dot semiconductor optical amplifier assisted Mach-Zehnder interferometer[J]. Journal of Lightwave Technology, 2013, 31(12): 2029-2035. DOI: 10.1109/JLT.2013.2263251
[18] GAYEN D K, CHATTOPADHYAY T. Simultaneous all-optical basic arithmetic operations using QD-SOA-assisted Mach-Zehnder interferometer[J]. Journal of Computational Electronics, 2016, 15(3): 982-992. DOI: 10.1007/s10825-016-0854-x
[19] KOTB A, ALAMER F A. Dispersion on all-optical logic XOR gate using semiconductor optical amplifier[J]. Optical and Quantum Electronics, 2016, 48(6): 1-10.
[20] HU Z, XIANG B, XING Y. Optical gain depending on both size fluctuations of quantum dots and temperature in InGaAs/GaAs QD-SOA[J]. IEEE Transactions on Electron Devices, 2017, 64(9): 3683-3689. DOI: 10.1109/TED.2017.2722383
计量
- 文章访问数: 3
- HTML全文浏览量: 1
- PDF下载量: 5