HTML
-
相移干涉数字全息术主要利用光电探测元件(如CCD、CMOS等)代替全息记录干板来记录全息图像,然后在计算机上模拟光的干涉过程对数字全息图像进行重建,从而实现全息技术从数据采集到重建全过程的数字化。相移干涉数字全息技术以光的波长为计量单位且具有全域、无损的特性,在物体表面形变测量、细胞分析、无损检测及光学元件缺陷检测等领域得到广泛应用[1-5]。光学相移干涉的基本原理是在两束相干光,即物光和参考光之间引入有序可控的相移,当物光或参考光的相位发生变化时,干涉条纹的强弱分布也随之发生相应的变化;在此过程中用光电探测器如电荷耦合器件(charge coupled device, CCD)逐步采集对应的干涉图,并将其转化为数字信号后按帧存储在计算机内;然后由计算机按照特定的相移干涉的波前重建算法由采集的数字干涉图样解出物光的复振幅分布。根据所需相移次数不同,可将相移法分为两步相移法[6]、三步相移法[7]和四步相移法[8]等。显然,随着相移次数的增加,所采集的干涉图像和耗费的时间也随之增加,这也是2步相移法较之其它相移法优势所在[9-10]。虽然传统的两步相移法与四步相移法相比,节省了近一半时间,但其算法和光路结构也相对复杂。2006年,MENG等人提出了一种两步相移干涉法,只需要两幅干涉图和一个单独的参考光强度分布来进行相位测量[11]。该方法要求参考波的平均强度比物光波最高强度高两倍,且随着物光和参考光相对强度的变化会对全息重建结果产生不同影响[12]。LIU和POON证明了相位成像可以用两个干涉图和参考波的估计而非测量来完成[13],其后又对该算法进行了优化[14],此算法虽然可以很好地去除零级像和孪生像,但计算原理较为复杂而且计算量较大,且使用压电陶瓷器实现相移操作不便[15]。
本文中所述的两步相移干涉数字全息算法,主要利用两个相同的CCD放置于距离像平面后的不同位置,同时采集不同位置的两幅干涉图以缩短采集干涉图像所需时间,然后利用光学相移单元在参考光中引入π的相移后再次采集两幅干涉图,并根据光学传递函数的性质得到相移前后干涉图间的数学关系,从而解出物光的波前分布,这样只需一次相移就可实现相位重建的目的。以微透镜阵列作为待测物体,验证了本文中提出的两步相移算法的可行性。
-
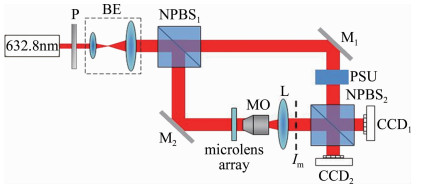
图 1为两步相移干涉数字全息系统示意图。系统的主体为一个Mach-Zehnder干涉仪,其中,光源为一个波长为632.8nm的He-Ne激光器。He-Ne激光器发出的光束经过透振方向沿竖直方向的偏振器P后入射扩束器(beam expander, BE),经过扩束器BE扩束后的光束由消偏振分光棱镜(non-polarizating beam splitter, NPBS)NPBS1分为参考波和物光波。在参考波光路中,放置一个光学相移单元(phase shifting unit, PSU),用于调节参考光的相位。在物光波光路中,一个微透镜阵列(microlens array,MA)作为待检测物体放置于4倍显微镜物镜(microscopic objective, MO)的前焦平面上。通过待测物体的物光波经一个由显微镜物镜MO和消色差透镜L组成的显微放大系统放大。Im为像平面, 放大后的物光波和参考波合束于消偏振分光棱镜NPBS2。型号相同的两个CCD,即CCD1和CCD2分别放置于距像平面为D1和D2的位置,用于采集对应位置的干涉图样。CCD型号为DMK 23G274, 分辨率为1600pixel×1200pixel,像素尺寸为4.4μm。
CCD1与像平面(x0, y0)的距离为d1。设Od1为物光波O(x0, y0)在自由空间中传播d1距离后的复振幅分布,可表示为:
式中,${\mathscr{F}}\left\{ {} \right\}$和${{\mathscr{F}}^{ - 1}}\left\{ {} \right\}$分别表示傅里叶变换和逆傅里叶变换。Gd1为自由空间传递函数,表示为:
式中,ζ和η为频域空间坐标,λ为激光波长。设平面参考波为R,则在d1位置处的干涉强度分布A11可表示为:
在参考光中引入π的相移后,d1处干涉图样的强度分布A12可写为:
则由(3)式和(4)式可得:
同时对(5)式两边进行傅里叶变换可得:
为简化计算,假设平面参考波R的复振幅为1。根据(2)式,可知Gd1的复共轭Gd1*和G-d1相等,再将(1)式和(2)式代入(6)式可得:
同理,通过位于距像平面(x0, y0)为d2的CCD2上所记录的两幅干涉图也可以得到如下式所示关系:
由(7)式×G-d1-(6)式×G-d2可解出待测物体物光波O(x0, y0)的频谱分布, 如下式所示:
式中, G-d1, G-d2, Gd1-d2和Gd2-d1分别为在距离-d1, -d2, d1-d2和d2-d1的自由空间传递函数。对(9)式进行逆傅里叶变换, 可以解出待测物体物光波O(x0, y0)的复振幅。这种方法不仅有着和传统四步相移相同的相位重建效果,而且只用了一半的时间。与传统相移算法类似[16-19],通过上述方法解出的复振幅分布仍然是包裹的[20],作者使用最小二乘法对包裹的复振幅分布进行解包裹,就可得到物光波的真实相位分布。
-
为了验证上述理论的可行性,首先使用MATLAB对其进行模拟验证。图 2为模拟的待测物体的振幅分布和相位分布。图 3中I11, I12, I21和I22为按照(3)式和(4)式等生成的数字全息干涉强度分布图。其中I11和I12为D1=61mm时生成的干涉强度分布图,且I11和I12之间有π的相移;I21和I22为D2=66mm时生成的干涉强度分布图,I21和I22之间相移同样为π。

Figure 2. Simulation of objects to be measured a—amplitude distribution of the simulated object to be measured b—phase distribution of the simulated object to be measured

Figure 3. The interference intensity distribution a—D1=61mm and phase shift is 0 b—D2=66mm and phase shift is 0 c—D1=61mm and phase shift is π d—D2=66mm and phase shift is π
按照上面所述方法对模拟出的干涉强度分布图进行相位重建,可得到图 4。对比图 4和图 2b中相位分布可知, 两步相移干涉全息算法确实能够实现相位重建的目的。
为了进一步验证两步相移干涉全息术的有效性,以微透镜阵列作为待测物体重建其相位分布。微透镜阵列为THORLABS公司生产的刻在紫外融石英底板上的熔融石英凸透镜阵列,型号为MLA300-14AR-M。
在实验过程中,由于光的衍射,干涉图应靠近物体像平面采集,因此,将光学相移单元放置于参考光路,且将两个CCD靠近合束棱镜NPBS2放置。作者将CCD1和CCD2垂直于光路放置,其与物体经显微放大
系统所成像的距离分别为47mm和52mm。由于实验所用的激光束并非理想的平面波,所用的光学器件也会引入附加残余相位,因此, 需要在不放置待测物体时采集干涉图重建系统的残余相位分布,实验结果如图 5所示。

Figure 5. Interference images collected by CCD a, b—residual phase images collected by CCD1 when the phase shift is 0 and π c, d—residual phase images collected by CCD2 when the phase shift is 0 and π e, f—the interferograms collected by CCD1 when the phase shift is 0 and π g, h—the interferograms collected by CCD2 when the phase shift is 0 and π
图 5a和图 5b分别为相移为0和π时CCD1所采集干涉图,图 5c和图 5d分别为相移为0和π时CCD2所采集干涉图。把微透镜阵列放置于显微放大系统的物平面,继续按要求采集4幅干涉图,结果如图 5e、图 5f、图 5g和图 5h所示。图 5e和图 5f为相移为0和π时CCD1所采集干涉图,图 5g和图 5h分别为相移为0和π时CCD2所采集干涉图。按照本文中所述的相位重建算法,经过拟合重建,当D1=47.2mm和D2=52.15mm(即ΔD=D2-D1=4.95mm)时对图 5a~图 5d的相位重建结果最好,如图 6a所示。在相同位置对图 5e~图 5h进行相位重建,重建结果如图 6b所示。图 6a是系统的残余相位图,图 6b是包含系统残余相位时微透镜阵列的相位分布图。

Figure 6. Preliminary phase reconstruction results a—residual phase diagram of the system b—phase distribution of microlens array including residual phase of the system
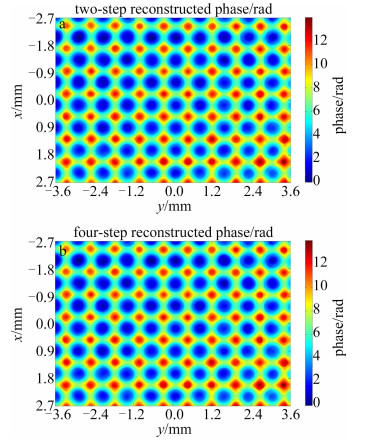
在包含系统残余相位的微透镜阵列相位分布图中除去系统的残余相位可以得到微透镜阵列的真实相位分布,结果如图 7a所示。为验证相位重建结果的有效性,同时使用四步相移法对微透镜阵列的相位分布进行重建,重建结果如图 7b所示。对比图 7a和图 7b可知,两种方法所得相位重建结果相一致,由此可知本文中所用的两步相移算法可以有效的实现微透镜阵列的相位重建。

Figure 7. The final result of phase reconstruction a—phase reconstruction result of two-step phase shift b—phase reconstruction result of four-step phase shift
实验中系统测量误差来源主要有:光学系统误差和相移误差。其中光学系统误差主要是透镜引入的像差可能引起干涉图样的变形,此外透镜对焦的不准确也会造成图像变形,使用高质量的傅里叶变换透镜可以有效减少此类误差。相移误差主要在于相移单元相移的准确度以及实验环境引起的干涉条纹晃动,提高相移单元相移的准确度以及在CCD采集干涉图时使用降低噪声的功能能够减少此类误差。
-
从理论模拟和实验结果可知,通过将两个相同CCD放置于不同位置同时采集物光波在不同衍射距离的干涉图,同时使用光学相移单元在参考光中引入特定的相移π,并根据光学传递函数的性质可得干涉图之间的函数关系,最终实现相位重建的方法是可行的。这种方法不仅结构相对简单、操作方便,而且比四步相移节约了一半以上时间,可以快速有效地实现透明物体的相位重建,理论模拟和实验结果都表明了这种方法的可靠性和有效性。

 Map
Map





 DownLoad:
DownLoad: